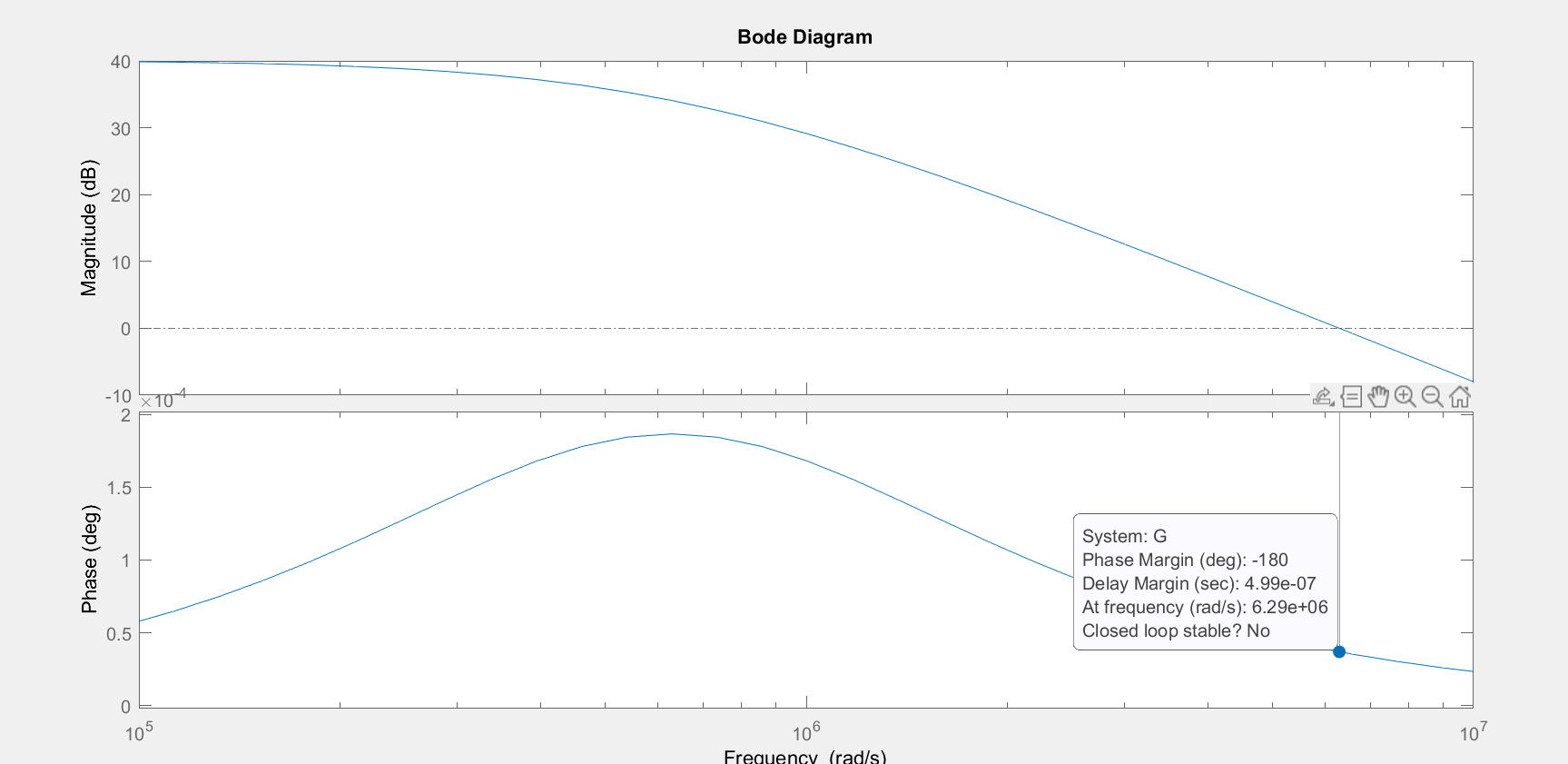
The relationship AB=1 will be applicable only at a specific frequency. For instance, following is the bode plot for a circuit I have and as can be seen, the barkhausen criterion is met only at 6.3MEG radian/s. But at frequencies lower than that, the system should be stable right? So why do we declare the whole system unstable?
3 Answers
Let's assume you have a system which is unstable at certain frequency as you say. Let that frequency be for ex. 1 Hz, so the system oscillates at 1Hz.
Unfortunately practical systems often do not offer a way to utilize the system properly at other frequencies. That's because often there's no practical way to filter out the 1 Hz oscillation from the system output signal nor to prevent the system wasting energy to keep the oscillation going on. The system may also get saturated and drift to some irreversible state when the oscillation amplitude gets high enough. Think for ex. an aircraft stabilizer program which makes the plane to swing at 1Hz. The pilot probably cannot feel any happiness due the fact that his plane is perfectly stable at 0,5 Hz or 2 Hz.
Thus we have used to think instability as a binary property. It exists or not.
But is the filtering always impossible? No. Most of us probably know how to construct a self-oscillating switch-mode voltage regulator or temperature controller. It can behave acceptably at low frequencies because we have a way to filter the oscillation out of the output signal.
But at frequencies lower than that, the system should be stable right? So why do we declare the whole system unstable?
A feedback system is unstable if it can freely oscillate at one or more "particular" frequencies. At any one of those "particular" frequencies, negative feedback becomes positive feedback and, the system is unstable.
You can't arbitrarily note that it might oscillate at such and such a frequency and then declare that it is stable below that frequency. It's still unstable. It's like saying a book is blank except for the words; it has no value or usefulness.
Because the whole system is oscillating at the Barkhausen frequency making all frequencies unusable.