Calculate current flowing through each of the resistors in the following circuit: [closed]

Question

Closed. This question needs details or clarity. It is not currently accepting answers.

Want to improve this question? Add details and clarify the problem by editing this post.

Closed last year.

This post was edited and submitted for review last year and failed to reopen the post:

Original close reason(s) were not resolved

I'm not sure how to go about this, but the answers are below

Where are you stuck? We don't answer homework questions without some evidence of effort. The answers given are sort-of correct. — Spehro 'speff' Pefhany, Commented May 13, 2023 at 20:28
This looks like a homework question, and we don't do homework on this site. If you specify exactly what you don't understand, I'm sure someone will help you. For a start, using Kirchhoff's Laws write down the 2 equations for the left and right hand loops letting current i1 and i2 flow through the 10 and 20 ohm resistors respectively. You now have 2 simultaneous equations in i1 and i2 you can solve with simple algebra. — Peter Jennings, Commented May 13, 2023 at 20:36
@AntonioDeAngelis - Hi, Thanks for trying to help. However: (a) You made a suggested edit (which was wrongly approved - I have reversed that) which put an answer from you as an update in the question. That is never correct etiquette on Stack Exchange. (b) The question is currently closed as it's a homework-type question and they get special treatment here - a solution attempt by the OP is required. So although I realise it can be frustrating when we have a solution, it cannot be posted as an answer on a closed question. Please answer non-closed questions :) TY — SamGibson, Commented Jun 5, 2023 at 18:25

Andy aka · Accepted Answer · 2023-05-13 20:34:59Z

0

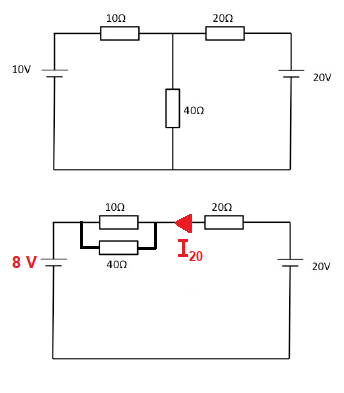
Here's a hint that simplifies your circuit in order to find the current through the 20 Ω resistor: -

Of course you could solve it with superposition but it's easier this way IMHO.

Once you have \$I_{20}\$ you can then reapply that current to your original circuit
And calculate the voltage at the common node of the three resistors That then gives you \$I_{40}\$ quite automatically
Once you have \$I_{20}\$ and \$I_{40}\$, and \$I_{10}\$ is easily revealed

answered May 13, 2023 at 20:34

Andy aka

473k29 gold badges383 silver badges839 bronze badges

\$\begingroup\$ It was actually quite easy, I'm not an EE but for some reason, it's on my uni course, thanks for any help provided. I realised I was overthinking a bit... \$\endgroup\$
– Xeocas
Commented May 13, 2023 at 20:52

Add a comment |

1 Answer 1