I am a fresher and have been introduced to a basic electronics course at college.
Recently our professor was introducing us to opamps and was referring to negative feedback as a rectification to any temperature changes in the amplifier.
Here is a schematic of a simplified opamp under -ve feedback:
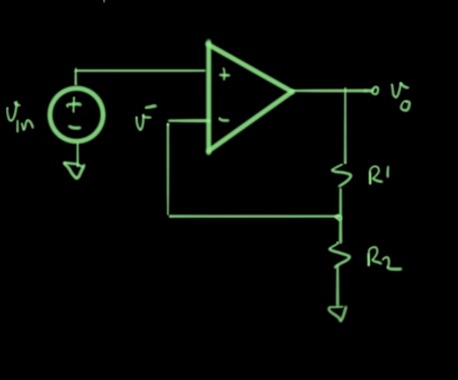
Now our professor did Voltage gain calculation for a Dc input source:
Let the gain of the amplifier be "A".
$$ V_o= A(V_{in} -V_{o}(\frac{R_{2}}{R_2+R_1})) $$
We could write this since no current enters the amplifier due to \$\infty\$ (ideally) input resistance.
On rearranging terms, we get $$ V_o= \frac{AV_{in}}{1+\frac{AR_2}{R_1 +R_2}} $$
now since A>>1 we get:
$$ V_o= V_{in}\frac{R_1 +R_2}{R_2} $$
Now we can do all this for an AC source as well and algebraically the only change we get is \$V_{o}\$ is replaced by say \$V_{in}sin(wt)\$ where \$w\$ is the frequency of small signal input.
But If I consider the negative feedback logic i.e. if \$V_{in}\uparrow\$ \$\implies\$ \$V_{o}\uparrow\$ \$\implies\$ \$V^{-}\uparrow\$ \$\implies\$ \$V_{o}\downarrow\$.
Which contradicts the fact that \$ V_o= V_{in}sin(wt)\frac{R_1 +R_2}{R_2} \$ can be possible by the above algebra(if it doesn't please explain) what mistake is there in my understanding? kindly explain..
Thank you
