This is an unorthodox answer, but under the circumstances, it seems reasonable. A brief explanation: user contacted me separately via e-mail; subject turns out to be this question, although along a somewhat different angle. In any case, perhaps others will find the explanation helpful.
Below, quoted text is the OP, and unquoted is my response. It has been edited to hopefully show the flow of discussion. (Image sources are assumed OP originals; I only checked the photos with Google Lens.)
Initial Question
I saw a few posts of yours on EEVblog and Stack Exchange and I would like some clarification on some points.
Specifically this post;
https://www.eevblog.com/forum/projects/emi-reduction-brushed-dc-motor/msg1253919/#msg1253919
I would like to reduce radiated emissions from common mode noise in an automotive application using what you've described here but am confused how it should be implemented. I will be providing DC outputs from an electronic sub assembly to other devices, perhaps motors or other subassemblies. So my device will have leads that could be long that connect to external devices and return, so I believe they will act like antennas.
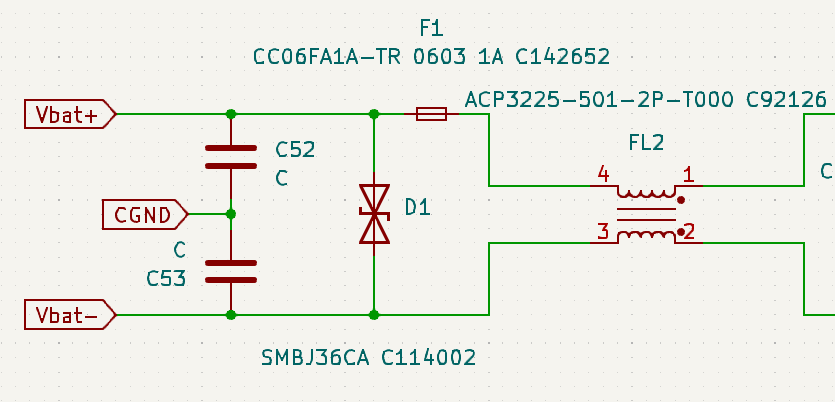
I believe you describe the use of MLCCs to do balanced decoupling where the capacitors are shunted to ground, in automotive applications this is the chassis.
Now In CISPR25 they describe that the power negative rail is actually directly connected to the ground plane during testing and commonly the negative rail of the battery is connected to the chassis in vehicles.
Supposing that you have this connection, will this filtering method still work? Here is how I assume it will be connected.

My Response
Afraid I don't understand the question. I suppose Vbat is the DC side, and LISN is coupled to plane as described. The right side is then the EUT side. But then there is no load, just two capacitors, one of which is shown connecting between grounds which seems redundant. Why are there two lines crossing through the LISN, is it a one-wire 5uH type, or two together, or..?
If your device is common-chassis (one-wire power), then there is no DM/CM, only normal mode, and you only need to filter that one connection with respect to the local chassis connection.
If your device has other connections, you need to ensure those aren't carrying noise as well. But no hint of these is shown on the diagram at all, so I am unable to correct further.
OP Response
Thanks for replying, I'm sorry about the poor diagram. Instead of describing it I'll show what it would look like in real life, but I think it would be two of the 5uH LISN types. The test is for this CISPR25 radiated emissions.
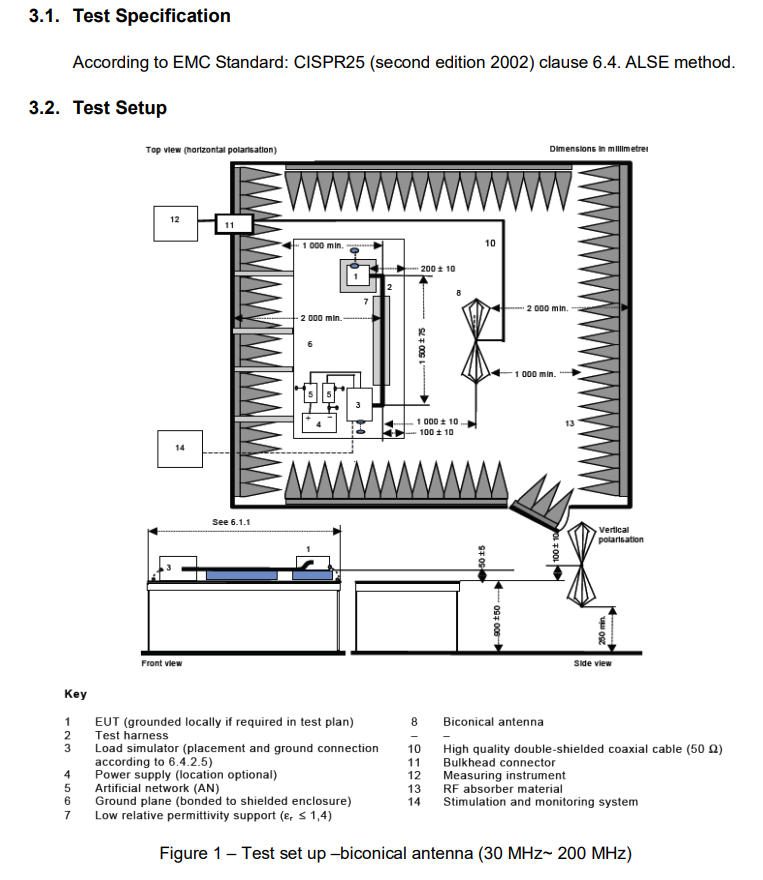
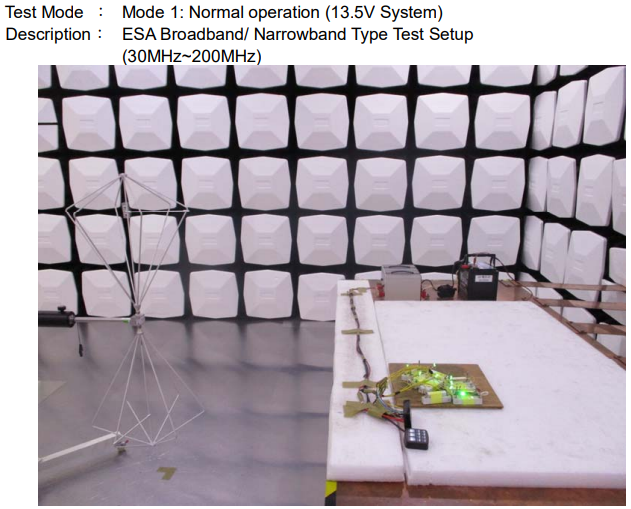
It appears that there is a negative return lead used in this test. There is also a dummy load connected.
I'm new to this so I don't know what these terms mean but is this image below is what common-chassis looks like when doing conducted emissions?

[Ed: appears to be from: https://www.ti.com/lit/ml/slyp780/slyp780.pdf?ts=1686822101756 ]
If your device is common-chassis (one-wire power), then there is no
DM/CM, only normal mode, and you only need to filter that one connection
with respect to the local chassis connection.
So in all circumstances where the chassis and battery negative are connected one doesn't have to worry about common mode noise? While the normal mode noise can be thought of as equivalent to how we think about differential mode noise?
That would also mean that there would be no way to use the balanced MLCC to decouple common mode noise because there are only two power lines and not three, right?
My Response
Not quite. The galvanic connections are irrelevant at RF. Don't see wires and nodes -- see inductors and transmission lines.
In EMC standards, there is often the concept of a "enclosure port", which represents the common mode currents, or sum of interactions (whether near-field as induced E/H, usually with common mode impact too; or far-field (radiating) waves), due to the bulk of the EUT itself, usually the enclosure -- chassis. If the enclosure is plastic, then consider the PCB within.
If you have a chassis-grounded application, then that chassis /will/ be metal, and /will/ be bonded to the ground plane. Which will be the closest representation of the real application (bonded to the automotive chassis), so is the best way to test.
The concept of a "port" at all, is also very useful, and necessary to understand EMC practices, as well as RF design.
Consider in DC analysis, we can simply trace the currents through wires, and that's that. Analysis is trivial, relatively speaking.
Suppose we define an arbitrary surface cutting through a circuit: the total sum of currents through the surface will be zero. In particular, wires have all the currents, and there is zero current elsewhere. We really only need to identify the wires, and add up their individual current flows.
This is much harder to do at RF, because the current is not localized to wires crossing through a surface. There are displacement currents through insulators and open air, and induced voltages (magnetic fields) that will not push currents through our cutting surface, but which nonetheless carry power across it.
Instead, let us make a combined assumption and stipulation: suppose the circuit is embedded within a good conductor. This conductor shorts out and absorbs all fields that would otherwise cross into free space. Space is no longer free. Suppose space itself were solid metal.
If we create a cavity within this "solid metal universe", we are guaranteed to have zero (or, asymptotically zero anyway) interaction with anything nearby in space to that cavity, but disconnected from it.
That is, we only get interactions by connecting cavities together, and wiring circuits within them.
This means we can continue using a circuit analogy: that is, constructing a graph (schematic) of the circuit, or a sparse matrix (node or mesh analysis) -- in which only connected elements need be considered.
We can avoid full 3D field analysis, while extending our simple analysis tools to much higher frequencies.
The fundamental element of connectivity in this scenario, then, is a single wire, surrounded by some insulation, surrounded by the "solid metal universe". In other words, basically a coaxial transmission line.
When we draw a cutting plane across a transmission line, we find equal and opposite currents flow, between the signal (core), and surrounding metal (shield). There is no current outside of the local area (alternating current drops off exponentially with distance into solid metal, so we really don't need a very wide cutting surface to measure essentially all of the shield current.)
Likewise we measure a voltage between the core and shield conductors, at least to the extent that the voltage is consistent around this circular cross-section*.
Combined with the Telegrapher's Equations, it happens that we can fully describe the waves propagating on the line, through this cutting plane, strictly based on the current and voltage measured at this point.
We have defined a port.
*This will be the case for frequencies where the TEM00 mode applies. Eventually, high enough frequencies will experience waveguide modes, and different propagation (velocity and impedance) characteristics apply. If we confine our analysis to TEM00 (low frequency, ordinary transmission line behavior, wavelength >> width of transmission line), we can treat transmission lines as the simple one-dimensional kind that are shown in undergrad EE courses.
Taking this observation back to our basic analysis tools, we find we can continue using traditional idealized schematics, so long as the elements within a given sub-circuit are close enough together that signals are basically instantaneous between them (that is, the circuit is point-like, zero-dimensional). And we can then fill in any real delays (connections between point-like sub-circuits) using transmission lines (TLs), which are one-dimensional wave structures.
Thus we can accommodate real propagation delays in a mostly-0D circuit, avoiding a full 3D field simulation.
We might not even need waves at all: we can often approximate TLs further, e.g. AC steady-state as reactance or an LC network, or transiently when waves can propagate as events, say with switching edges.
An ideal port, then, for circuit analysis purposes, is: a two-terminal component, which has some current flow from one pin to the other, and some voltage drop between them.
A port need not have a definition of the /relation between V and I/ -- that would represent some kind of component. The port is a level of abstraction above that.
Put another way: an ideal port is a perfectly floating two-terminal element.
Resistors are generally good approximations of a 1-port network.
An ideal transformer is the simplest 2-port network, where the ports simply connect 1:1, but are otherwise perfectly isolated from each other -- or at whatever ratio really, I suppose.
Notice the stipulation that the port's two terminal currents match: there is zero common mode, a port is an ideal transformer, it can float perfectly on top of any voltage.
A network can have any number of ports, with some (V, I, and time/frequency) relationship between all of them.
For N-port networks, in general, we define a coupling matrix between ports. Which includes self-coupling (a port to itself -- basically, its input impedance). We also need not be restricted to LTI networks (basically, RLC+TL networks, and small-signal amplifiers), but those are of course easier to measure and model!
Alternately, we can define all ports as common-ground, in which case one pin is defined as ideal ground, and the other is some voltage and current. The trivial common-ground 2-port then is simply two ports in the same location: a zero-length transmission line. (It could also be an ideal autoformer of some ratio, but the 1:1 case seems more deserving to be called "trivial".)
The latter definition most closely represents the case for coaxial transmission lines, and circuits over ground plane, and is also the case relevant to EMC purposes.
By splitting a circuit into idealized sub-circuits, joined by common-ground ports, we can analyze the ideal sub-circuits with traditional tools (nodal analysis or whatever), and employ TLs to express real delays where needed. Because everything is common-ground, we don't have any common mode or stray-field interactions to worry about, and everything is measured perfectly at the end of a transmission line.
Also, note that [well behaved*] transmission lines are resistive when terminated with R = Zo, so the line length is unknowable from a port looking into it. This gives us great freedom with how we connect networks and cables, as long as they're all terminated appropriately of course.
*Dispersive lines need not be resistive when terminated. Or put another way, Zo can be complex. It turns out this is the usual case (ultimately because, for the usual TL construction, series and shunt losses don't match), but not to a severe enough degree to worry about it for lab purposes.
Now, more about the EMC application.
A LISN is a device to separate DC power and RF signals on one side, and couple them together to an EUT. An EUT which, in general, may not have an intimate connection with the plane (notice the foam dielectric elevating the pictured EUT above the plane!).
The problem with the pictured device is, there can be common-mode where the PCB (local ground plane) voltage changes one way, and /both wires/ change the opposite way. The board has capacitance to its surroundings (or more complex wave relationships at higher frequencies, but something about that size, capacitance would be dominant up to the ~100MHz range), therefore there can be unbalanced currents between the two leads, and two one-line LISNs are required to fully characterize it.
It's not enough for one line to simply be tied with ground, because that wire link is flying through space, coupling with fields. The most immediate effect of which is, at low frequencies (again, up to 100s MHz give or take), the wire acts as an inductive loop. Thus, there will be an LC resonance, between the PCB's capacitance to plane, and the wire link's inductance between PCB and plane, which act as a parallel resonant tank, in series with the power line to the PCB.
Whether the configuration shown was rightly deemed adequate for the test, or is representative of final application, I don't know.
To explain the "solid metal universe" thought experiment a bit more:
Note that currents only flow locally, within a shielding layer of the surrounding metal. AC drops off exponentially with distance through solid metal.
Suppose we remove all the metal outside these local shielding layers, returning it to free and open space again.
As long as this coating of metal surrounding our circuit cavities remains contiguous and thick enough, nothing much has changed. We can have arbitrarily high shielding effectiveness (attenuation) by using an arbitrarily thick layer.
And we might only be concerned with so-and-so amount of shielding in a given circuit, so we can adjust the shielding thickness to suit.
Thus, we can relax the "solid metal universe" rule, and turn it into a matter of shells. Put another way: we've grown the perimeter of our circuit, and in that added space, filled in with solid metal.
Put another way: we've started with a "solid metal universe", and created a bubble of "outer space" and grown it until it just wraps around our circuits (minus some remaining thickness). Now a great many wave modes can propagate freely in that surrounding space, but it still doesn't interact with our circuit (at least, within some degree of approximation).
And we can relax the rule still further, and perhaps say the metal shielding doesn't need to perfectly surround everything, we might permit gaps up to some nominal length and width. This reduces attenuation still further, but perhaps nearby fields are small to begin with (there might be multiple levels of shielding), or coupling is small for other reasons such as mismatch loss (e.g. a capacitor bypassing a power supply input/output).
In this way, we can go from a "solid metal universe", to gradually hollowing out the, well, the rest of the universe of free space (as we know it) around the embedded circuit, and ever so gradually and carefully opening up the circuit to that free outside space, in only the careful and specific locations and amounts we have exposed.
This is a lot of words to describe something that, well, would probably save many words with a few diagrams -- but also, to be very precise about how to think about and approach this subject, deserves a few words.
This description also relies a fair amount on topological transformations, which, if you also aren't comfortable with, probably won't help much as a means of imagining what it looks like, how surfaces would be permitted to move, and not. (Or also if you don't have a strong imagination in the first place, which happens sometimes, and is all the more reason to fault me for not including figures...)
Also, I don't know how common or popular this sort of explanation is, going from a solid conductor, to hollows of circuits embedded within, to mostly free space as shielded circuits, and so on. I don't think I've heard an explanation start this way, so, that might be points against it. It sounds good enough to me, but it has been a long time since I learned ideas like topological transformation, long enough I've forgotten what confusion they may create. Maybe this is a terrible didactic route, I don't know...
OP Response
Not quite. The galvanic connections are irrelevant at RF. Don't see wires and nodes -- see inductors and transmission lines.
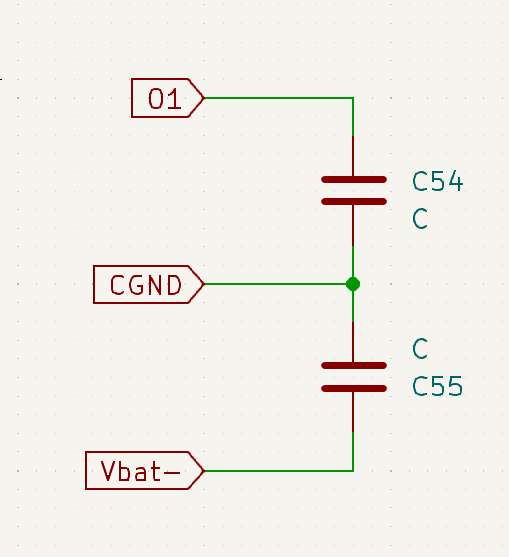
In that case, since galvanic connections are irrelevant at RF, I assume that there will be capacitive coupling between the reference ground plane and the circuit. This means two things to me, the first is that I should worry about common mode currents and the second is that the balanced filter can be useful.
This is my current mental model.
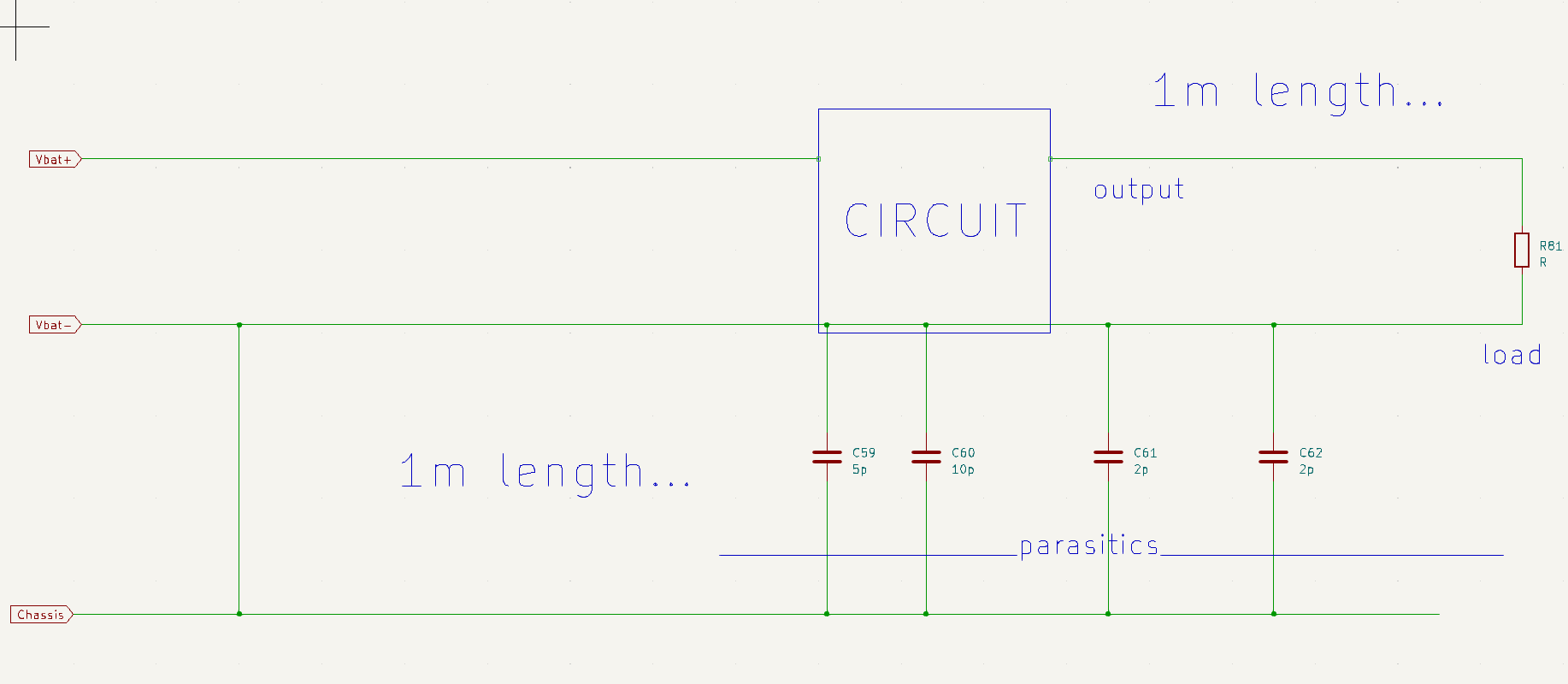
It is odd though, usually the model for common mode noise I think about assumes that the third line (e.g. earth) is galvanically isolated from negative. If there is a direct connection wouldn't that mean there is a shorted path which is much lower impedance for the higher frequency to take? Perhaps if the parasitic capacitance was orders of magnitude smaller there may be some frequencies that would like to transmit themselves as displacement currents but that would be way beyond my area of interest especially since I'm only concerned with EMI up to 6GHz.
On the rest, I remember some parts of the EM theory from school but I would need diagrams to make sense of that much theory at once. It would be much more helpful to get answers to specific practical questions.
Specifically, would it help to shunt each output line to chassis with a capacitor in order to reduce common mode noise from the parasitic to chassis even though the chassis is connected to battery negative?
My Response
Note that where you label "1m length", there are distributed inductors in both wires. Which therefore define a resonant frequency for their total equivalent value against the total equivalent capacitance, and thus a resonant frequency and impedance.
On the rest, I remember some parts of the EM theory from school but I
would need diagrams to make sense of that much theory at once. It would
be much more helpful to get answers to specific practical questions.
The problem is that "specific practical questions" will be framed incorrectly, and thus answers (drawn from and justified by theory) will be inconclusive in that [incorrect] perspective; or they won't be understandable or applicable at all without theory.
I strongly encourage looking up resources on the subject. Anything from regular searches, to textbooks and real course content. There are numerous open and online courses these days; the quality might vary, but there is nothing limiting a mind sufficiently committed to studying these topics in great depth!
[At this point I had identified and mentioned this question; OP confirmed the connection.]
OP Response
Note that where you label "1m length", there are distributed inductors
in both wires. Which therefore define a resonant frequency for their
total equivalent value against the total equivalent capacitance, and
thus a resonant frequency and impedance.
Thank you. I remember that for the distributed line we will have something like this, this is a model for a 1.2m power feed line.
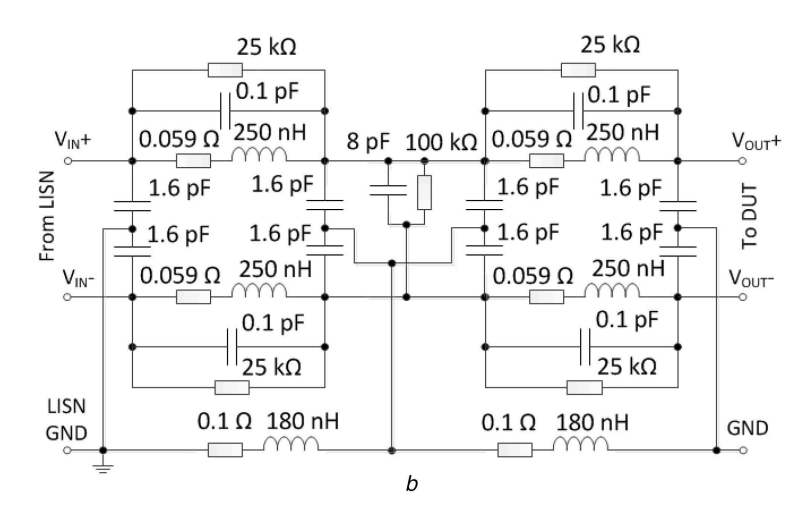
Then it becomes clear that there are series inductances in all the lines including the reference ground plane, and at higher frequencies this can be a noticeable impedance meaning the negative and the ground (e.g. chassis) aren't going to be equal voltage. From my take this means it will be worth attempting to use the decoupling method.
On theory and practice, of course one needs to learn the theory well but the path to science is treacherous. Bogatin often says a good answer now is better than a great answer later and I think that's true. I'm making my way through Ritchey's Right the First Time but one problem I tend to find is that I will read theory and textbooks and they are illuminating but when I actually have a question or a confusion I can't seem to find the answer anywhere, probably because it is based in a simple misunderstanding but it can't be assumed that someone asking for help hasn't exhauted their current resources. On this question alone I read about 12 published papers on impedance balancing.
Thanks for the help, I'm more clear on what is happening now. I'm going to go ahead and try impedance balancing and remove the capacitors to see if there is a difference.
[End of e-mail conversation]
I don't know the provenance or applicability of the above model; it would seem OP couldn't have created it, but perhaps a coworker or test engineer was able to. Whether it's specific to OP's current testing, or obtained from unrelated work, isn't clear. Likewise, whether the above photos are related to the present question, or only provided as supporting material, isn't clear.
I would also like to add some remarks about the nature of EMC and problem solving here:
- EMC is a holistic subject. There is no detail too small to leave out, and the scope is always much, much broader than newbies suspect!
- If theory is not directly understood, then taking any general proposal seems ill advised. That is, simply putting capacitors or inductors here or there, without understanding exactly their effect on overall system response -- their relationships with system parameters -- is as likely to make things worse as it is better.
- It's tempting to ask a question like "is this input filtering circuit okay?", but such a question is impossible to answer without also knowing the impedances the circuit is surrounded by, what the source levels of noise are (is it filtering a buck converter or a linear amplifier?), what tolerable emissions levels are (mV? µV? At what frequency(ies)?), and what other ways noise could get out (it doesn't matter a damn how much you filter the power supply, if there's two other connections that happen to provide a direct path from the same noise source!).
- For my part, personally, the only way I can be confident answering an EMC question, is to have the EUT literally in my hands, with a test setup, and equipment ready to measure it (and with whatever supporting documentation, schematics, layout, BOM, assembly and harness drawings, etc. to facilitate the inevitable modifications). The output of which, will be an extremely narrow conclusion: one specific set of steps to take, or changes to make, for that particular item, for that particular test setup. Needless to say, for the amount and detail of work to arrive at such an answer, a work/consultation contract would be indicated.
- Conversely, what I would not provide, with any kind of confidence, to someone lacking theory on the topic, is general suggestions, as far as an analysis of the problem space and a set of selection rules with which to choose components, or ways in which the design can be mutated successfully. That is, an assessment of the space around the solution space. The number of constraints is simply far too vast to create a checklist or flow chart; and it's unlikely (in my humble estimation) to be followed accurately enough (let alone accurately created in the first place!). So, almost any advice I do provide on this subject, is to encourage a theoretical understanding, upon which to base further analysis or answers.
- In contrast, generic advice ends up looking very random; sometimes one kind of filter is recommended, other times the complete opposite. Few people have truly mastered EMC, and yet, many [read: more than are plausibly masters] do indeed offer generic answers to these kinds of poorly defined questions. And they're as much of a mixed bag as you would expect, given the above. Which leads to further confusion on the subject.
Indeed, even when multiple EMC masters weigh in on a question, and have correctly guessed missing context, they can still arrive at seemingly very different conclusions! What you are missing, is the large quantity of assumptions that have been made, both upon the initial guess/reply, and in mutual discussion between masters. It's something like two close friends discussing an inside joke, leaving you completely lost all the while -- there is a background of shared assumptions, which is rarely articulated, but which underlies the conversation. And, within that set of assumptions, multiple answers may indeed be correct, whether related by an underlying symmetry (even if the circuits or values seem unrelated), or by taking different approaches to the problem.
And, those assumptions are rarely articulated because, well, it leads to massive ramblings posts like this for one (upvote if you're still reading this?); but also those assumptions can be so deeply internalized that they aren't even made consciously anymore!
(For my part, I consider myself "pretty good" at EMC. There are still plenty of circuits, boundary conditions, etc. that I haven't seen in person, nor have an intuitive feel for; that, or I just miss some particular detail and end up going around in circles a while before finally challenging that earlier assumption. Whether that makes me a "master", is probably a matter of opinion.)