Reasoning used when solving current mirror circuit

Question

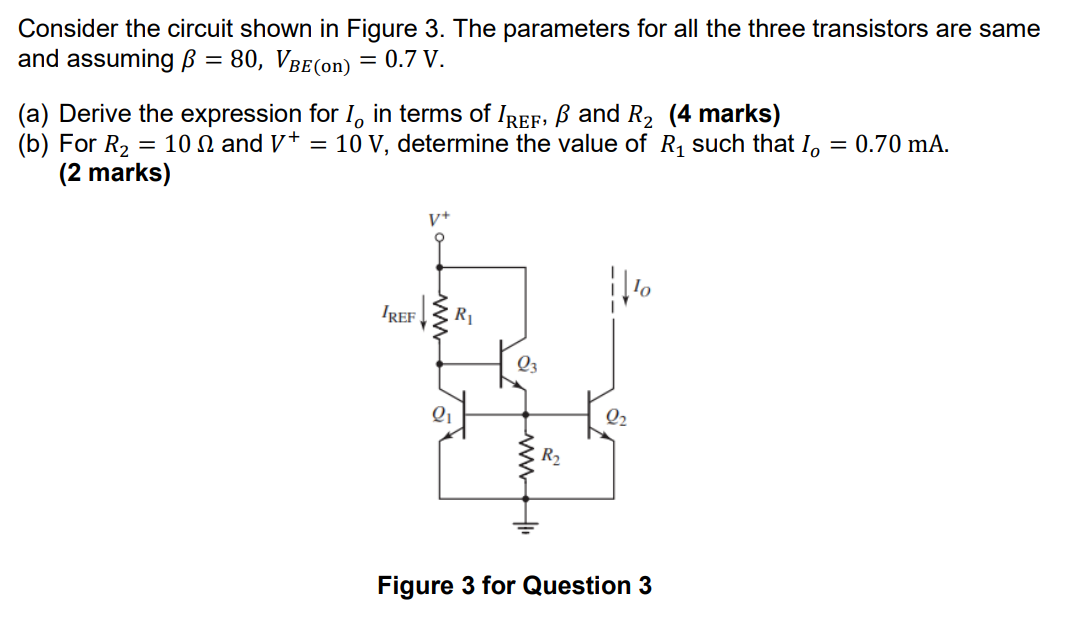
I'm confused by the reasoning used to solve part a) in this question and when solving current mirror problems in general. From what I've seen the common method is to say that \$I_{B1}=I_{B2}\$ since \$V_{B1}=V_{B2}\$ and the transistors are identical. However, in the question it also states that \$V_{BE ON}=0.7V\$ which implies a vertical line at \$V_{BE}=0.7V\$ on a \$I_{B}\$ vs \$V_{BE}\$ graph i.e. a diode I V curve. However, if this were the case then we can't be sure that Q1 and Q2 share the same base current since each could have any base current along the vertical ranging from 0 to infinity. Can someone explain why we can say that \$I_{B1}=I_{B2}\$?

Answer 1

Strict math and the approximative nature of "ON-state Vbe=0,7V" clash here. The maker of the problem tries to find those persons who intuitively can reject the strict math and think Vbe is not exactly 0,7V, it depends on Ib and that happens in the same way in Q1 and Q2. But it's still so close 0,7V that the calculation by assuming Ib1=Ib2 and Vbe=0,7V is useful. The found people are seen useful in future practical works.

Answer 2

Depending on the particular application a PN junction (diode or base-emitter junction here) can be thought (desired) to have:

• zero forward voltage (rectifier diodes)

• 0.7 V forward voltage (voltage stabilizers acting as low-voltage Zener diodes)

• nonlinearly changing forward voltage (log and antilog converters, current mirrors)

When two non-linear but mutually inversed converters (I-to-V log and V-to-I antilog here) are cascaded, the whole converter I-to-I is linear. This is the idea of the current mirror.

So the third case is valid here and not the second as advised.