1
\$\begingroup\$

I have come across this document https://www.ti.com/seclit/ml/slup386/slup386.pdf , where injection points for stability measurement are discussed in Fig.26. enter image description here

For point 3 it says: "Because the op amp has negative feedback, the inverting input of the op amp is virtually the same as the positive input, which is a DC reference voltage, VREF. VREF appears as an AC short, making the equivalent impedance looking forward almost zero."

I suppose (but am not sure) the "negative feedback" mentioned here is the local feedback of the error amplifier, i.e. just the compensation components. This is what makes the input impedance error amplifier almost zero, not the global feedback that includes everything (PWM comparator, drivers, filter,...).

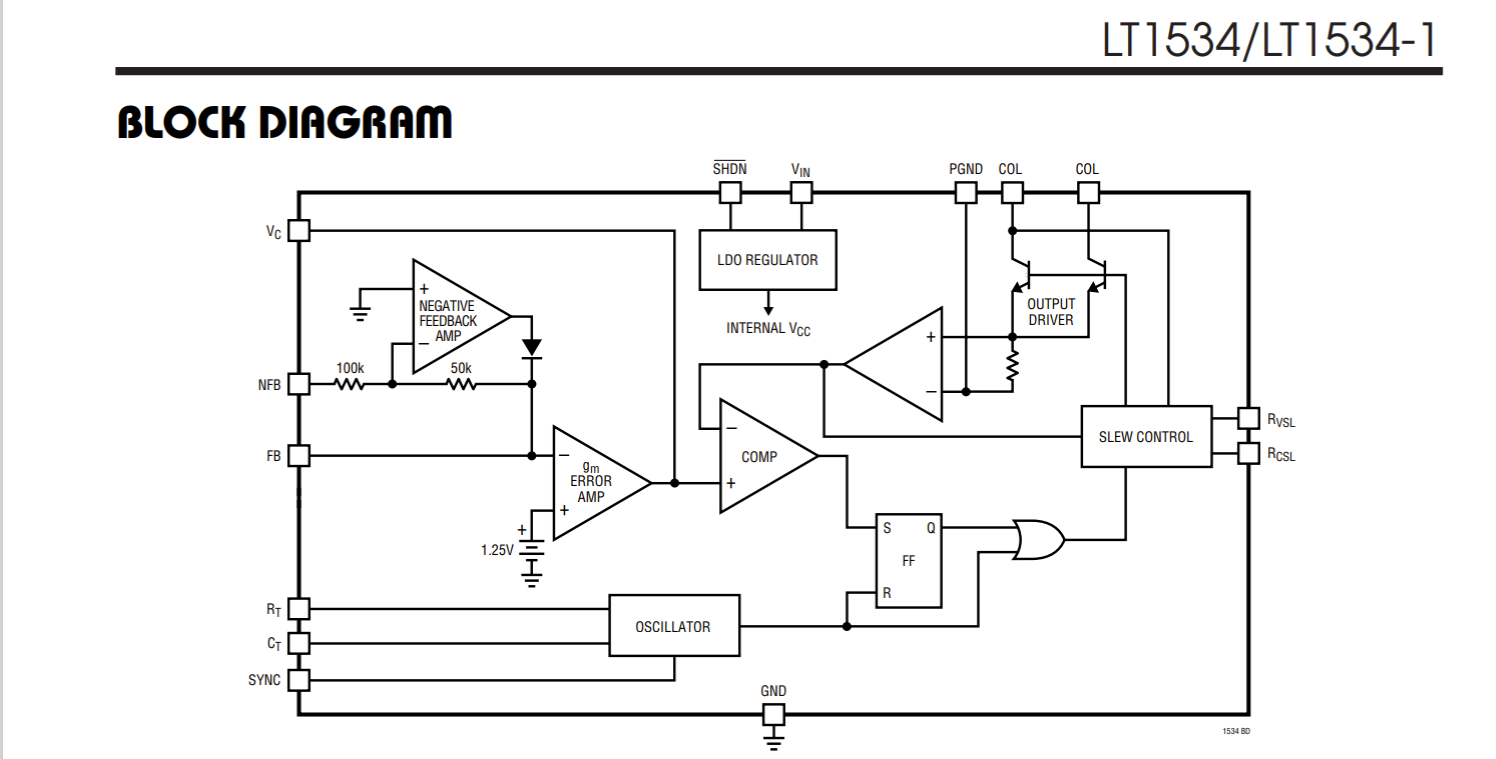
If this is true, could an injection point between the resistor divider and the FB pin be valid if the error amplifier was not an operational amplifier but an OTA, which has no local feedback (compensation components from output to ground)? And in the special case of LT1534 https://www.analog.com/media/en/technical-documentation/data-sheets/1534fa.pdf with its negative feedback amp: even with NFB pin disconnected, the negative feedback amp and its output diode would make the impedance looking into the FB pin either very small or very large and the injection point at FB would not be valid, correct? And even buffering the divider with an operational amplifier would not make its impedance much smaller than the impedance looking into the FB pin of LT1534.

EDIT: I overlooked the fact that for the LT1534 the negative feedback amp's output diode will be closed when Vfb = 1.25V, rendering the negative feedback amp irrelevant wrt impedance looking into the FB pin.

So my main question is: is the OTA's input impedance high because it has no local feedback (unlike an op amp, which has a local feedback via its compensation components)?

enter image description here

\$\endgroup\$
5
  • \$\begingroup\$ I believe you're right; between the divider and a gm amplifier wouldn't be a suitable injection spot since there's no virtual ground and the input is a high impedance point. \$\endgroup\$
    – John D
    Commented Sep 18, 2023 at 17:11
  • \$\begingroup\$ @JohnD Maybe this is a misunderstanding. My point was that I do want the input to be high impedance (compared to the divider) to make the measurement valid. However, I have doubts whether this is the case for an OTA (only) and an OTA with a negative feedback amp as in the LT1534. \$\endgroup\$
    – Hyp
    Commented Sep 19, 2023 at 5:35
  • \$\begingroup\$ You might be OK if your divider is low impedance, and the gm amplifier is much higher impedance for all frequencies of interest, but the LT part is a gm amp with negative feedback (so like an op-amp) and it looks like the input impedance will be 100 k, at least at low frequencies. \$\endgroup\$
    – John D
    Commented Sep 19, 2023 at 16:04
  • \$\begingroup\$ @JohnD Why 100 k? The only 100 k I can see is between the NFB pin and the IN- of the negative feedback amp but the NFB pin is disconnected in my question (usual usage of the LT1534 I would guess). \$\endgroup\$
    – Hyp
    Commented Sep 20, 2023 at 5:38
  • \$\begingroup\$ Sorry, looked at the block diagram too quickly, it's not 100 k. But still, if there's negative feedback around a gm amp then it can still "act" like an op-amp. \$\endgroup\$
    – John D
    Commented Sep 20, 2023 at 14:50

1 Answer 1

Reset to default
0
\$\begingroup\$

Not quite: the local components only deliver negative feedback at such AC frequencies as they pass -- of course. The DC loop is closed around the rest of the system. Together, they work to implement the op-amp feedback condition, -IN = +IN.

Therefore you can only inject AC transients into the loop by connecting in series with the compensation network.

By the same token, it doesn't work to inject in series with the compensation network of a gm amp, between OUT and GND. (There is no network between OUT and -IN in this case.)

Tweaking a gm amp would instead be done by injecting a current step in parallel with the output, or a voltage in series with the input in the usual way.

You may still see useful transient results, by such AC-coupled disturbances; but realize you're seeing only the differentiated (sort of) response, not the full step (or whatever) response. Similarly you can load-step test the output, or input, which gives reasonable information about the transient condition, but error trends towards zero over time, so you have a very poor measurement of loop gain this way.

Correct, the LT1534 works by disabling (rather, output saturating to 0V) the NFB amp when not in use.

\$\endgroup\$
9
  • \$\begingroup\$ Didn't you mean "divider network" instead of "compensation network"? Wrt the mechanism creating the feedback condition -IN=+IN: yes, the op amp compensation network closes the loop at AC frequencies and DC around the whole system. But I'd say it is only the compensation network that affects the input impedance, using the Miller effect, while the global DC feedback sets -IN=+IN by setting outside voltage going into the resistor divider. So the only input current path is through the compensation network. This path disappears with a gm amp, no current = inf impedance, a possible injection point. \$\endgroup\$
    – Hyp
    Commented Sep 20, 2023 at 8:47
  • \$\begingroup\$ Compensation components, in regards to this part, "I suppose (but am not sure) the 'negative feedback' mentioned here is the local feedback of the error amplifier, i.e. just the compensation components" \$\endgroup\$
    – Tim Williams
    Commented Sep 20, 2023 at 9:02
  • \$\begingroup\$ Miller effect doesn't go away simply because the feedback is carried on different components. The "global DC feedback sets -IN=+IN by setting outside voltage going into the resistor divider" is still precisely the same feedback mechanism, with the same result. \$\endgroup\$
    – Tim Williams
    Commented Sep 20, 2023 at 9:08
  • \$\begingroup\$ But where would the current go? It cannot flow into -IN of the gm amp, the compensation components are not connected to -IN either. I have tried and simulated an LT1534 SMPS now injecting a small AC voltage at different frequencies across a 10 Ohm resistor added between a feedback divider and the FB pin. This results in 20mV superimposed on 1.25 VDC on the FB pin, while the current into the FB pin is a nearly constant 250nA (corresponds to Ifb typ in DS). Dividing the voltage by the current in the graph gives me around 5 MOhm, so it looks like there no low impedance. \$\endgroup\$
    – Hyp
    Commented Sep 20, 2023 at 10:46
  • \$\begingroup\$ I wasn't talking about a gm amp, either. Your specific motivation (or interest in, or complaint with?) for this summing node impedance, seems to be irrelevant, so I've not paid attention to that part of your question. \$\endgroup\$
    – Tim Williams
    Commented Sep 20, 2023 at 10:55

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.