How to calculate receiver gain budget?

Question

My receiver has the following system architecture:

I know about the parts rules but I want to be specific because I am confused. I am using the AD831 as mixers, AD8367 as IF amplifier and LM386 as audio amp. Old, tried and trusted parts, no surprises with exception to impedance.

Input BPF has Z=50 ohm, mixers also have Z=50Ω, IFBPF is a 100 Ω, IFVGA is Zi=Zout=200 Ω. I don't understand what is Z for LM386 but I will assume it is a lot because input resistance claimed to be 50 k.

The maximum voltage gain of LM386 is 46 dB. The voltage gain makes me confused because normally we deal with the power.

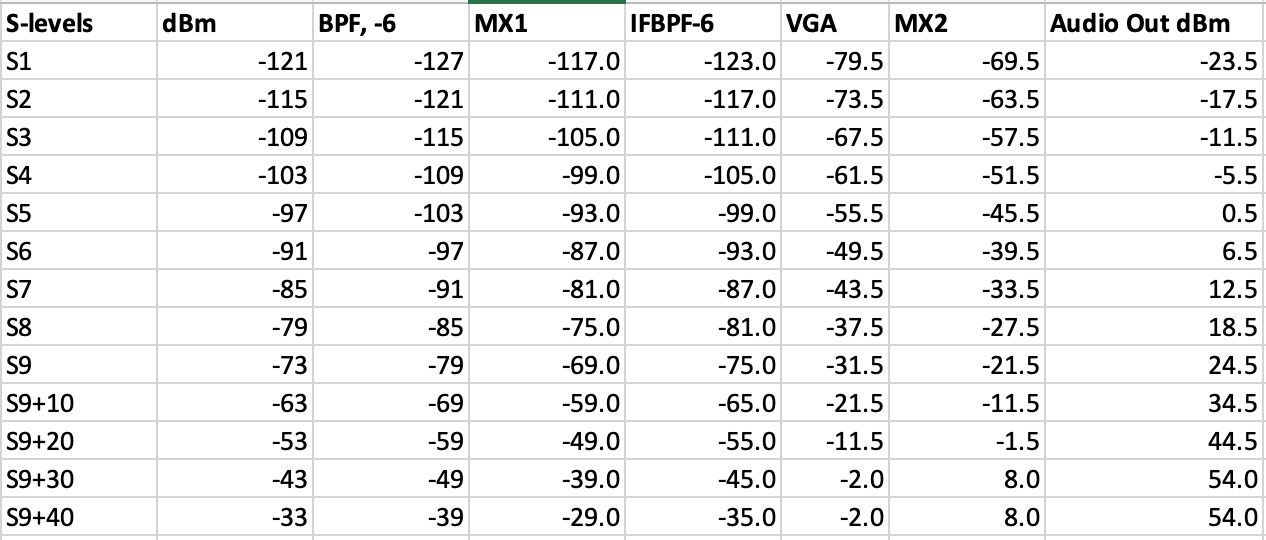
If we take these 46 dB and try to simulate signal chain we get the following results:

44 dBm and more seems to be crazy.

I do understand this is a voltage gain but what to use as a reference point? 50 kΩ?

For example, if we have -1.5 dBm at 50Ω then Vp-p = 0.266 V.

If we look at Vp-p=0.266 V with 50 kΩ input resistance then we have -31.5 dBm @ 50 kΩ.

46 dB is a gain of 200 times. So, 0.266*200 = 53.2 V @ 50 kΩ which is an impossible number for LM386. And none of audio amps is loaded with 50 kΩ output. Not even a valve PAs.

So this all makes me think that I should consider mixer output load and audio amp input to be taken as 2 parallel resistors so its common resistance is pretty much the same 50Ω.

If we do that we should use Vp-p = 0.266 V converted to -31.5 dBm @ 50 kΩ and amplified by 46 dB gain which I presume becomes 14.5 dBm at 4 Ω if we use 4Ω speaker and the amp magically transforms the impedance. This is 28 mW of electrical power.

Now, I tried to read about speaker sound pressure level and relation of this to the power of the amplifier. I figured that 1 W at 1 meter distance produces about 90 dB SPL. This is equal to a very noisy street.

If we look at 1W/28 mW = 36 which is about 31 dB. 90 dB SPL - 31 dB = 59 dB SPL which is quiet conversation.

This sounds a bit strange to me.

I believe that I missed something important and I would appreciate someone to correct my train of thought.

Answer 1

That's the problem; LM386 is simply an old audio amplifier IC with adequate quality.

It should not be analyzed using RF context and concepts like power in dBm, but just with regular audio context, which means it is simply an amplifier with voltage gain and ability to drive enough current (and thus power) to a speaker.

You can simply model the LM386 like an op-amp, or just a black box. Assume you have audio output from somewhere with low enough impedance to drive the 50k input impedance, in which case it can be assumed that it does not load the source much and could just as well have infinite input impedance.

Then it simply just has voltage gain and it has approximately zero output impedance to drive a speaker with that voltage.

If it helps, don't use dBm as LM386 input units or dB as units for LM386 voltage gain. The input is simply voltage, not power in dBm into 50k load, and voltage gain is simply 20 to 200 instead of 26 to 46 dB.

As the LM386 takes virtually no power in, and can push a lot of power to load, it has basically infinite gain if you think in watts and it makes no sense.

Otherwise your LM386 calculations are about correct - for example, 0.05 volts input RMS with gain of 20 will be 1.0 volts output RMS, and that into speaker with some ohms like 8 provides some amount of power into the speaker. And speakers do have sensitivity measured around 80-90 dB SPL when fed with 1 watt and measured at distance of 1 meter.

But your example of having about 0.2Vpp in, with gain of 46dB or 200, would output 40Vpp into 8 ohm speaker, and frankly that is impossible and makes no sense, as the LM386 supply voltage can't be that large. It would also be about 14Vrms and 8Vrms into 8 ohms would already be 8 watts.

Answer 2

The traditional formulas you see for RF cascades, such as for gain and noise figure, assume that all elements in the cascade have the same input and output impedances and are connected by interconnects matched to this impedance. This is so common, and so useful in so many circumstances, because it makes the math easy, that people often don't state that is an assumption being made. Which can make what you read confusing!

Trying to apply these formulas to cascades that include elements of mixed impedances. I.e. your audio amp, which has a relatively high input impedance and relatively low input impedance, is a common source of confusion. As you have realised it becomes easier to work in terms of voltages very often, rather than power.

Gain of RF components is measured with some particular source and load impedance e.g. 50 Ohms. The datasheet of the component will indicate the impedances used in characterizing the component. The gain when inserted into a circuit with different source and load impedances will be different.

For example, say we are driving an RF amplifier with a 50 ohm source, and it has a gain of 20 dB when driving a 50 ohm load. If we then connect that amplifier and source to a 10k Ohm load, the gain, Pout/Pin, will not be 20 dB, due to the large impedance mismatch now present at the load.

In your example, the effective conversion loss of your mixer will not be the datasheet value, which was measured with a 50 Ohm load, as the input of the LM386 does not present a 50 ohm load.

Practical RF System Design by William Egan gives a good treatment of mixed-impedance cascades as opposed to what the author refers to as 'standard cascades'. I am taking a moment to digest the mathematics at the moment, I'm not across it enough to give a numerical answer right now sorry.