How to use integration or differentiation to optimize frequency in achieving a specific phase angle?

Question

Question:

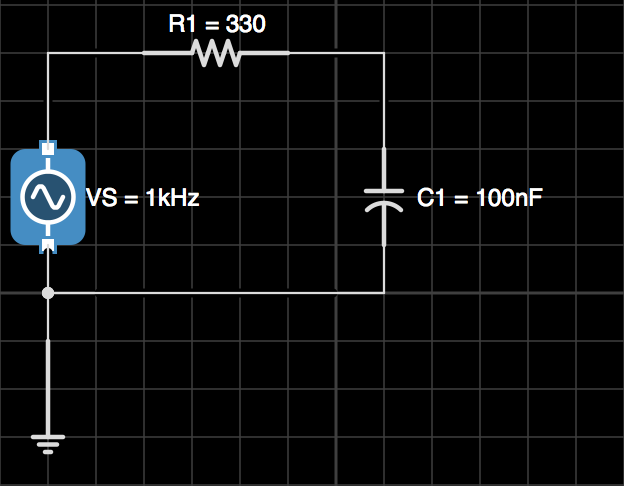
With source voltage kept constant at 8V peak to peak vary the frequency until the phase angle "theta" Vr referenced to Vs is approximately 45degrees. Record the Vpp value across R1 and the frequency at which "theta" is equal to 45degrees.

Situation:

For clarity I will call R1 the resistance in ohms and Theta Vr the phase angle.

I can approach this two ways from what I know. I could use my simulator and increase the frequency step by step. At each time I would have to figure the reactance and perform the following calculation to determine theta. This process would be very long to get an approximate answer for the frequency.

Theta Vr = ARCTAN(Xc/R1)

Xc = Reactance of the capacitor "ohms"

R1 = Resistance "ohms"

Theta Vr = "Theta" Phase angle of Vr referenced to the Source Voltage (VS)

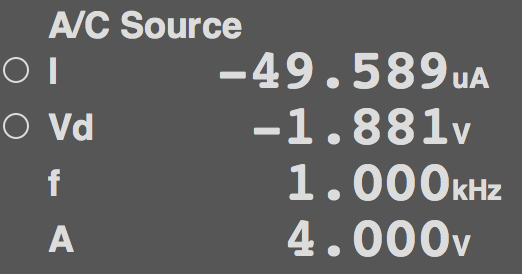
NOTE: With the source set to 8Vpp and frequency set to 1kHz Theta Vr = 78.27489 degrees. With the source set to 8Vpp and frequency set to 1kHz Theta Vc = 11.72511 degrees.

The other way I thought would be to derive a formula that resembles a optimization problem. Derivatives will solve for which the slope is zero. So it would seem I could approach this the same way when solving for the frequency.

Finally, lets say we want to change R1's value and repeat this procedure. It would seem that there has to be a generic expression where X is in ohms and Y is in frequency so this calculation is much less tedious.

Any ideas?

Here is a screenshot of the circuit for clarification.

Here are the parameters of which I set my AC source to.

Answer 1

The complex voltage phasor at the resistor is $$V_R=V_S\frac{R}{R+\frac{1}{j\omega C}}= V_S\frac{j\omega RC}{1+j\omega RC}$$ For the capacitor we get $$V_C=V_S\frac{\frac{1}{j\omega C}}{R+\frac{1}{j\omega C}}=V_S\frac{1}{1+j\omega RC}$$ For \$V_R\$ and \$V_C\$ to have a \$45\$ degree phase shift with respect to \$V_S\$, we need to have equal real and imaginary parts: $$\omega RC = 1\quad\Rightarrow\quad f=\frac{1}{2\pi RC}$$

Of course there's always a frequency-independent phase shift of \$90\$ degrees between \$V_R\$ and \$V_C\$.

Answer 2

If complex notation is a bit difficult to get your head around think of the problem in terms of a simple right angled triangle (a la Pythagoras) problem.

The 'real' component of the current is the one we get if we only consider pure resistance. (V = IR - Ohms law)

The 'imaginary' current is (always) at right angles to this 'real' current. The measured current (phasor) is the hypotenuse of the triangle.

To calculate its value we need to know the reactance (ac resistance) of the capacitor. That is inversely dependent on the frequency of the source and the size of the capacitor (in Farads) and can be easily calculated from the equation 1/wC , where w (sorry don't have an omega) is equal to 2 x pi x F .

For F = 1 KHz and 100nF (as per illustration) this would give a value of

1/((2 x 3.14 x 10^3) x 10^-7) or approx. 1k6 ohms

As has already been pointed out in the previous answer that you will get a 45 degree phase shift when the resistor's 'resistance' and the capacitor's reactance are the same ohmic value. So the only thing needed to do is work out the frequency when the reactance equals the resistance. (i.e. your general solution for theta = 45 degrees)

In this case R = 300 (given)

R = 1/(wC)

300 = 1/((2 x 3.14 x F) x 10^-7)

Re- arrange the formula to solve for F

F = 1/((2 x 3.14 x 300) x 10^-7) = 5,305.2 Hz

No need to guess!