Is the analysis of the following circuit correct?

Question

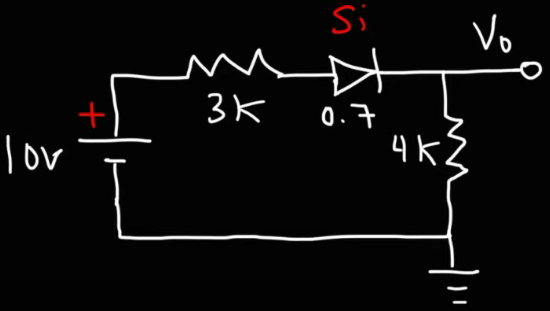
Watching this video I encountered an analysis of the following circuit:

The value of \$V_0\$ is computed as follows

1. As \$I=V/R\$ by Ohm's Law, we set \$V= 10V - 0.7V\$ and \$R=3k\Omega+4k\Omega\$, getting \$I = 1.33mA\$.

2. Again, by Ohm's Law we know \$V=IR\$. Setting \$I=1.33mA\$ and \$R=4k\Omega\$ we get \$V=5.32V\$, which is the value of \$V_0\$.

Is the reasoning correct? If the voltage at a point is to be measured in terms of the resistance in front of said point (as \$V_0\$ was measured in terms of the \$4k\Omega\$), would that not imply that measuring the voltage at a point in between the battery and the first resistor should be done as follows:

\$V=IR=(1.33mA)(3k\Omega)=4V\$

even though the voltage at that point should intuitively be \$10V\$?

Answer 1

Yes, this reasoning is correct, but you've overlooked one thing:

This is only correct because one side of the resistor is defined as being ground.

What you are actually calculating with V=IR is the voltage difference between the two terminals of the resistor.

Your second calculation is also correct--but it doesn't tell you that the voltage on the left of the resistor is 4 V above ground, it tells you that the voltage on the left of the resistor is 4 V above the voltage on the right of the resistor. Since you know that the voltage on the left of the resistor is 10 V relative to ground, this means that the voltage on the right is 6 V relative to ground.

This is consistent (taking rounding errors into consideration) with your calculation of Vₒ as being 5.32 V, because if you add up these voltages and that of the diode, you get 4 V + 5.32 V + 0.7 V = 10.02 V, where the extra 0.02 comes from how you rounded in your calculation of Vₒ.

Answer 2

If you see a node showing a voltage, then the voltage at that node is shown with respect to (w.r.t.) ground. Vo, for example, is shown like floating but the node's voltage is specified w.r.t. ground.

If you measure a voltage "across" a component then that's a different thing.

Vo in your case is the voltage on top end of the 4k resistor. But the resistor's bottom end is also ground. So, the voltage across the 4k resistor and the Vo node becomes the same thing.

The voltage drops across the 3k is 4V. This means that the "potential difference" is 4V. So the voltage to 3k's left-hand side, w.r.t. ground, and the voltage at the right-hand side, again w.r.t. ground, has a difference of 4V. The 3k resistor's left-hand-side voltage, w.r.t. ground, is 10V, obviously. And that at the right-hand side, is 6V, again w.r.t. ground. The difference is 4V which is inline with your calculation i.e. the drop across 3k.