Transfer function of a heating system

Question

I wanted to design a small temperature-regulated DC heater for the sake of educational purposes in the field of control theory.

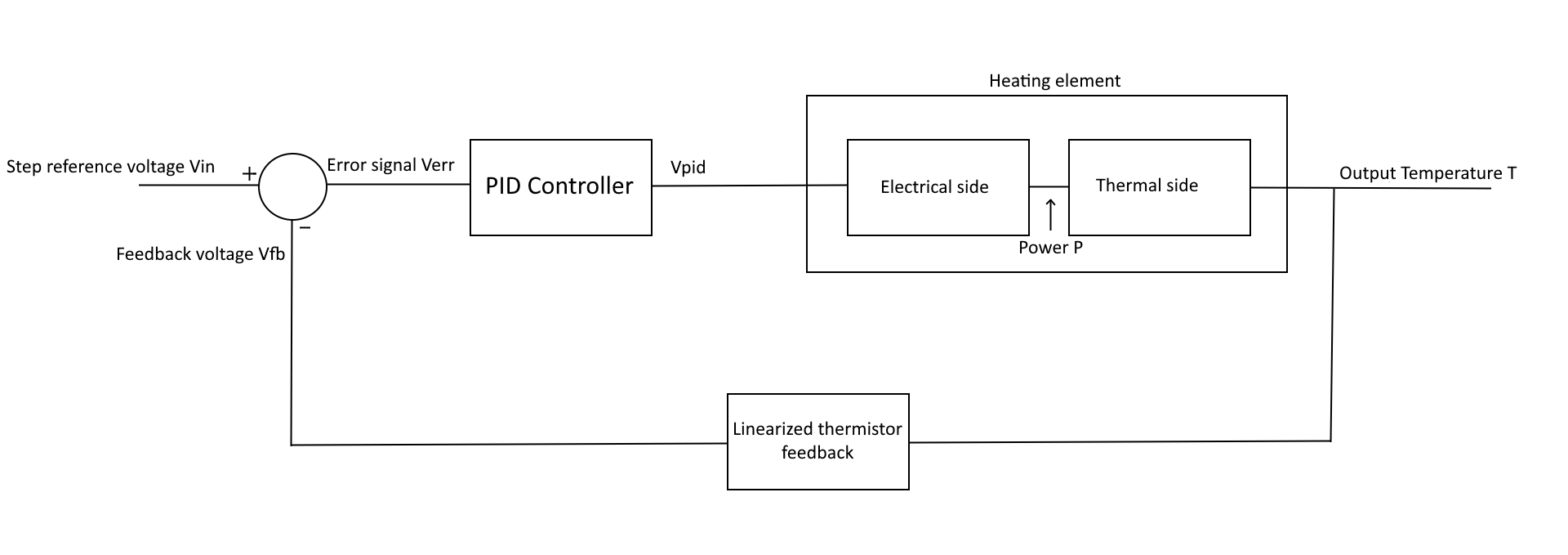
The idea is to heat up a resistive element through a buck converter of some sorts and to regulate that with a control loop involving a PID controller for the controller and a linearized thermistor for the feedback, like pictured in the following block diagram:

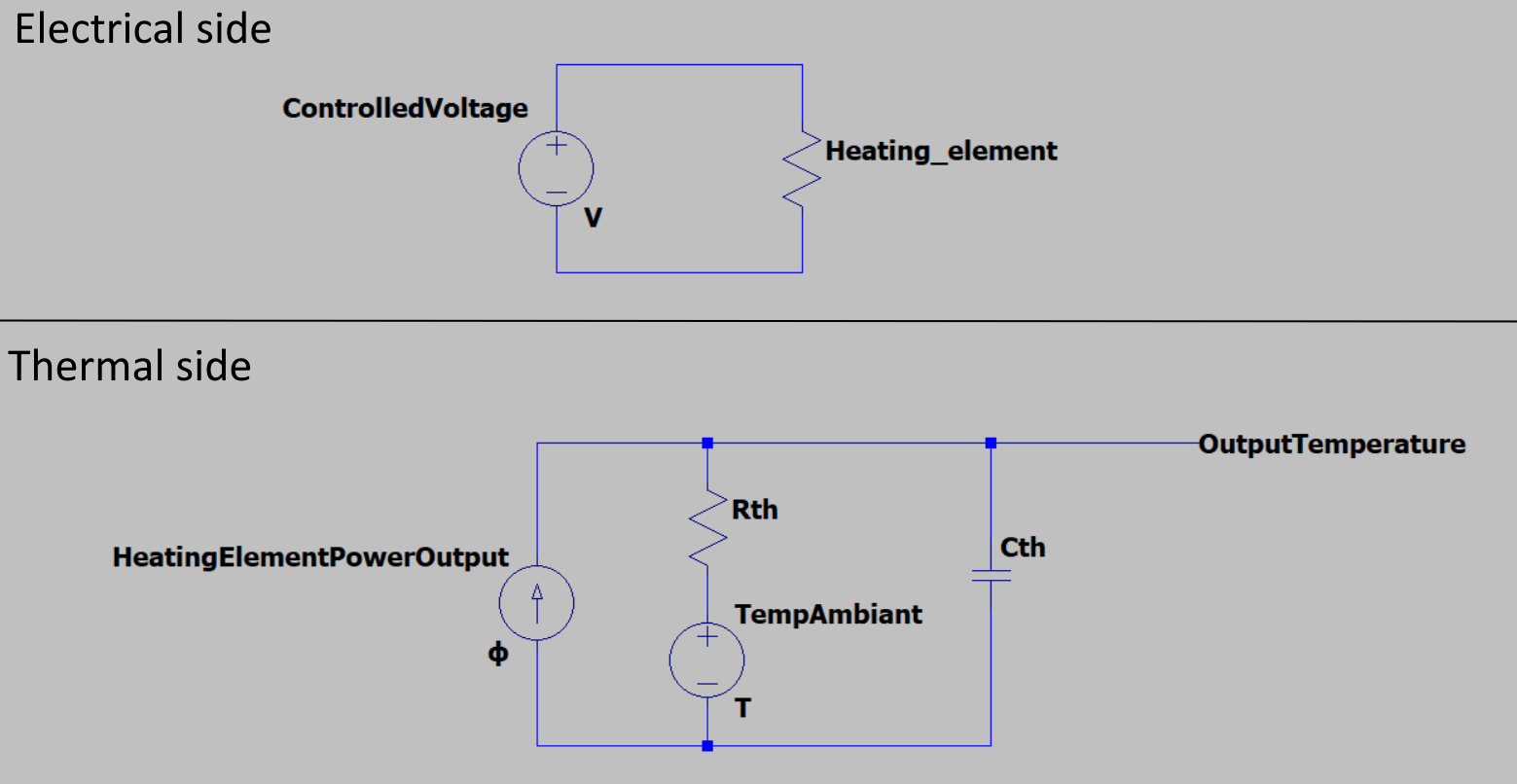
I naturally wanted to first analytically find the transfer function of that heating system which incorporates both electrical and thermal equations, the schematic of the system I came up with looks like the following:

Please note that for the sake of simplicity:

1. I am oversimplifying the electrical side, "ControlledVoltage" should eventually be driven by a buck converter but as this isn't related to my current issue I'll assume that it is directly proportional to the output voltage of the PID controller ("Vpid").
2. For the thermal side I'm using the thermal-electrical analogy to model the thermal behavior of the heating system through an electrical circuit

So, it should be easy to find the transfer function of the thermal side as it's nothing more than an equivalent RC circuit with the thermal flux ϕ as an input and the temperature of the heating system as an output.

However, when attempting to find the transfer function of the electrical side with the controlled voltage as an input and the dissipated power in the resistor as an output, I obviously find:

$$P = \frac{V^2}{R}$$

Which is a non-linear equation, which is also a huge problem since transfer functions are by definition linear time-invariant systems, meaning that I cannot use this equation to model my transfer function or that I need to find some way around it.

So my question might appear obvious now, how can I find the transfer function of my system? I am obviously not the first person in the world to attempt to design a regulated DC heater and as such probably also not the first one to stumble across this issue, is there some tricks to linearize the system, some equations I missed, something I simply didn't understand about transfer functions, or maybe an entirely different design philosophy I missed?

Please note that I am aware that I could build the system, find the impulse response experimentally, curve-fit it and eventually find the transfer function. But again, I'm doing this little project for educational purposes and I would greatly appreciate finding the transfer function analytically.

Any help would be greatly appreciated.

Answer 1

There are several ways to handle the non-linearity implied by the power being proportional to V².

• Linearise around an operating point. For small disturbances, power will be roughly proportional to voltage. However, this solves the problem only in the region of that particular point. This is a well used approach, often used to get a g_m figure for bipolar transistors at a particular bias point. However, you still need to make sure the system is stable at all heating levels, you will be powering on with the system cold, see the next point.
• Make the system stable at a range of gains. If you want the system to operate with the different gains you get from operating at high or low voltage, then you need to be very generous with your gain and phase margins, and tolerant of slower than ideal settling times over a range of settings. This can be very easy to do if you use Bode plots for design, you simply move the low-pass elements up, and the high-pass ones down in frequency, to give you an extended 6dB slope region in the middle. Aim for over-damping. With a heating system, depending on the tolerance of your load to an over-heat (for instance biological samples or soft plastics), you must have no possibility of an overshoot.
• As you have a heater, lose the buck converter, use simpler PWM, and now you have a linear transfer function between PWM setting and heater output power (power = full_power * PWM_fraction). Take advantage of the fact that a heater time constant will be seconds to 10s of seconds, so the PWM can be very slow. My oven controller uses a 20 seconds PWM period. I had a precision lab hotplate that managed quite happily with a two seconds period.