I have some questions about the link between the intuitive explanation for why an antenna radiates (based on this video) and the Maxwell equations.
Q1. The video starts by saying that "an inductor with AC supply does not radiate" because the field-lines are just fluctuating around the source. In the animation, the field lines expand and contract around the source without detaching from it.
I don't understand this statement. Formally, I don't see any difference between Maxwell Equations for an antenna and an inductor with same AC supply. In both cases, If I take a point in space, I believe the homogeneous helmholtz equations are valid. The Ampere and Faraday Equations are identical. The only difference I can see is on the physical length of the circuit compared to the wavelength. I'd say that an inductor utilized for non-radiating purposes, the field-lines will detach much far away compared to one utilized for radiating purposes. Am I wrong?
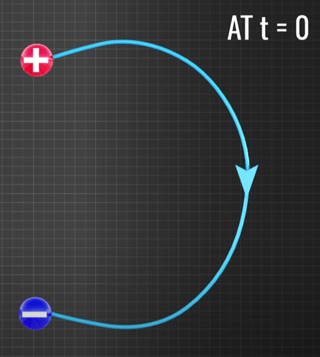
Q2. Now it considers a dipole with AC supply. At time 0, charges start with maximum separation, zero speed and maximum acceleration. All the past time is supposed to be static so their electric field lines are the same as those of a regular static dipole.
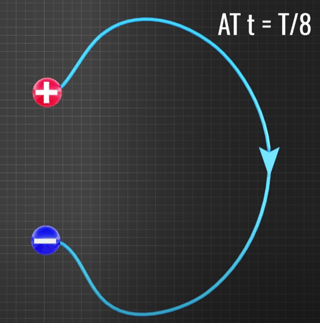
At time 1/8 of period T, the charges have less separation, more speed, less acceleration. The field lines are not exactly the same as a static dipole or even a constant-speed dipole with same charge separation because of the retardation of field propagation (I can see this by looking at Jefimenko's Equations).
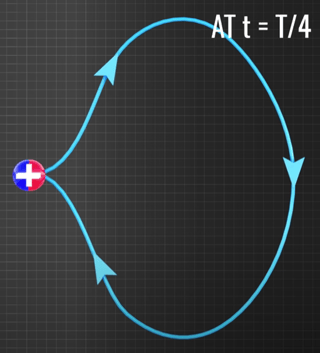
At time 1/4 of period T, the charges have zero separation, maximum speed, zero acceleration. The video says that field-lines detach from the source at this time.
Let's formalize better this last event. I'd say that zero separation between charges means there is no net charge in that volume of space. That means that the divergence of electric field (homogeneous medium) must be zero. This means that the past diverging lines cannot exist any more, so these pre-existing lines must detach from the source.
Am I wrong?
Q3. From this video it appears that for an antenna is crucial that the electric field lines detach from the source. I really don't understand this. This animation from MIT shows no detaching of field lines in a dipole because we are too close to see the detaching. But there still is a time-varying electromagnetic field in these points of space. Why do we want the field lines detach?