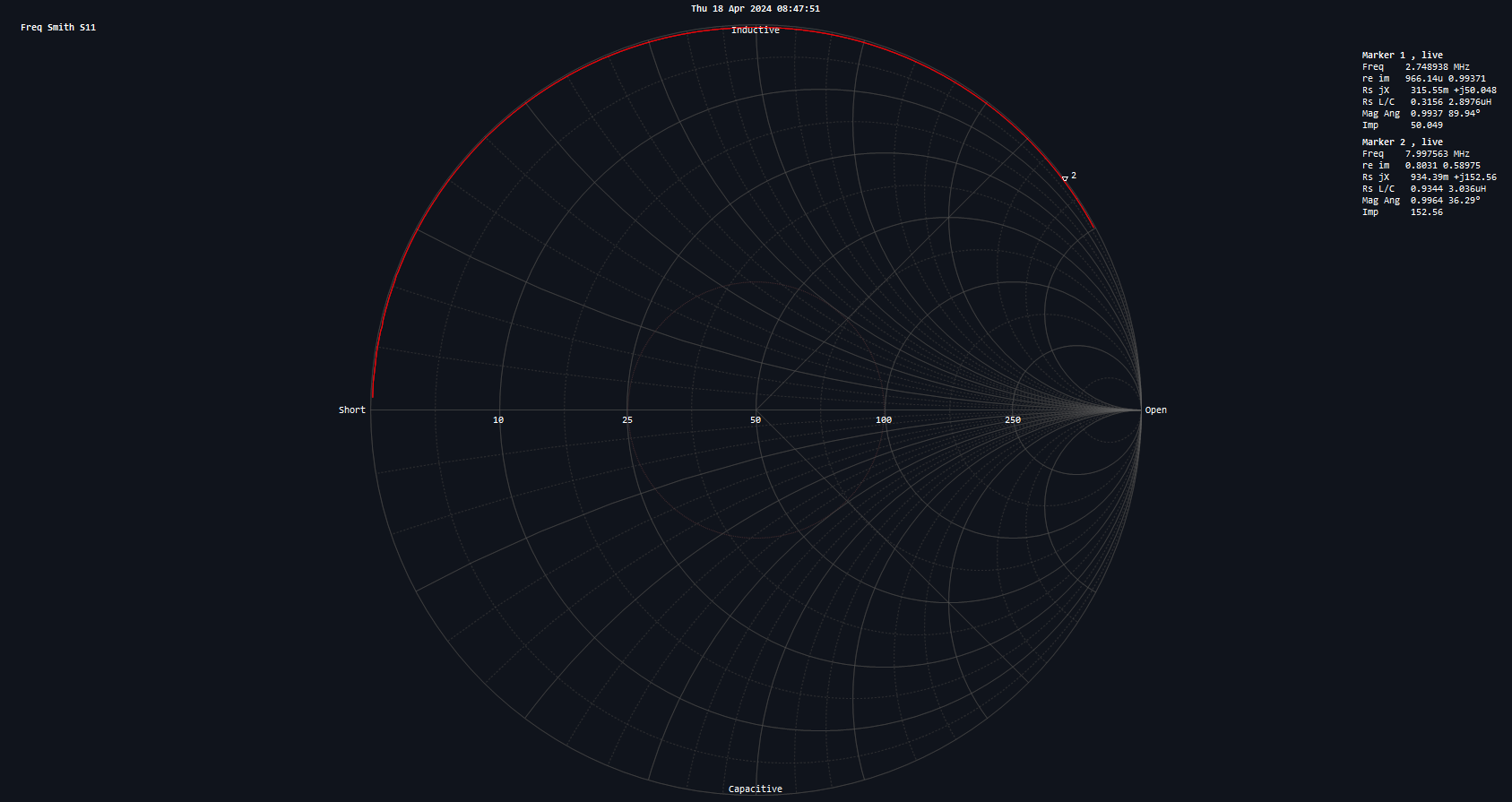
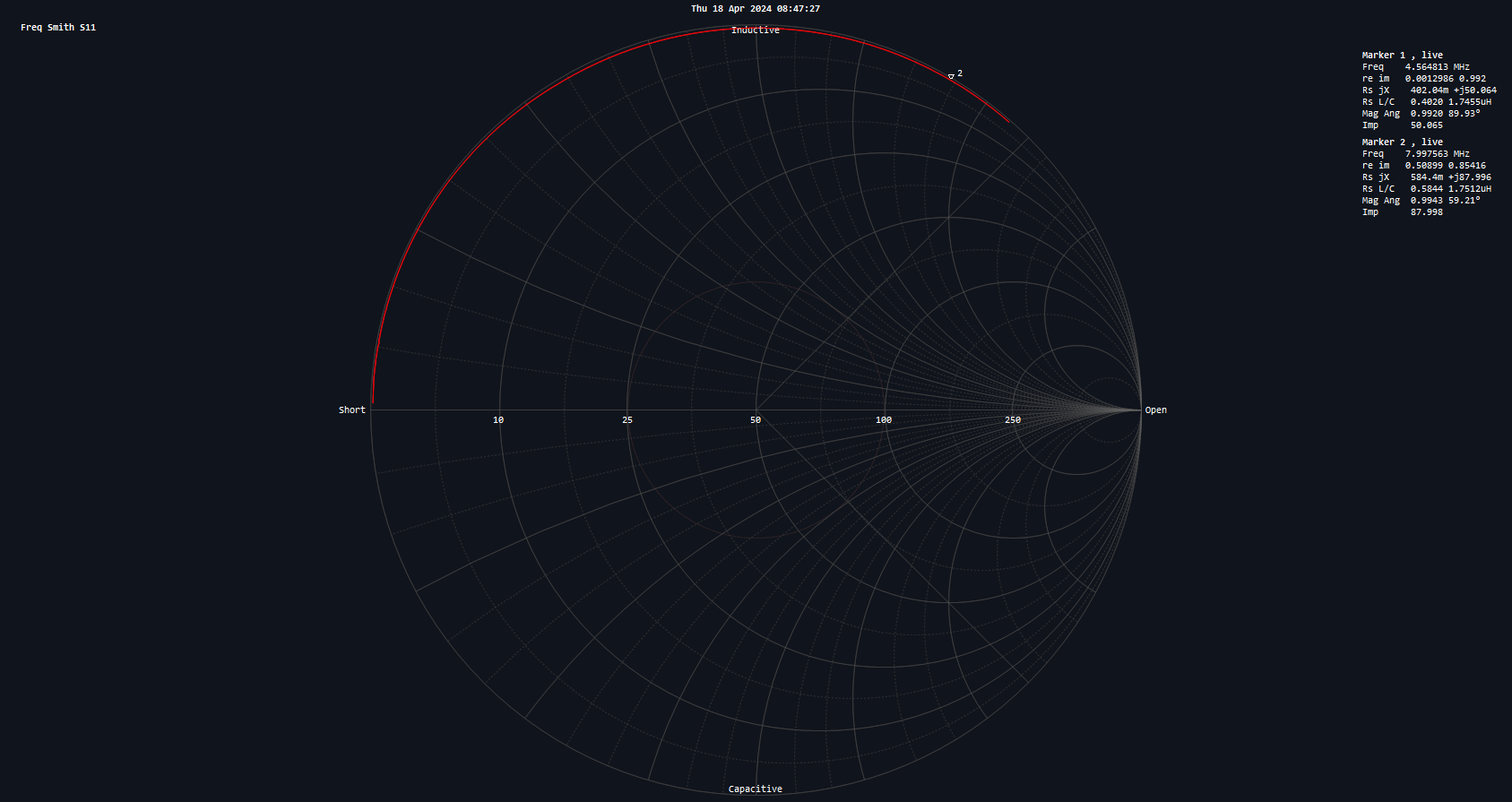
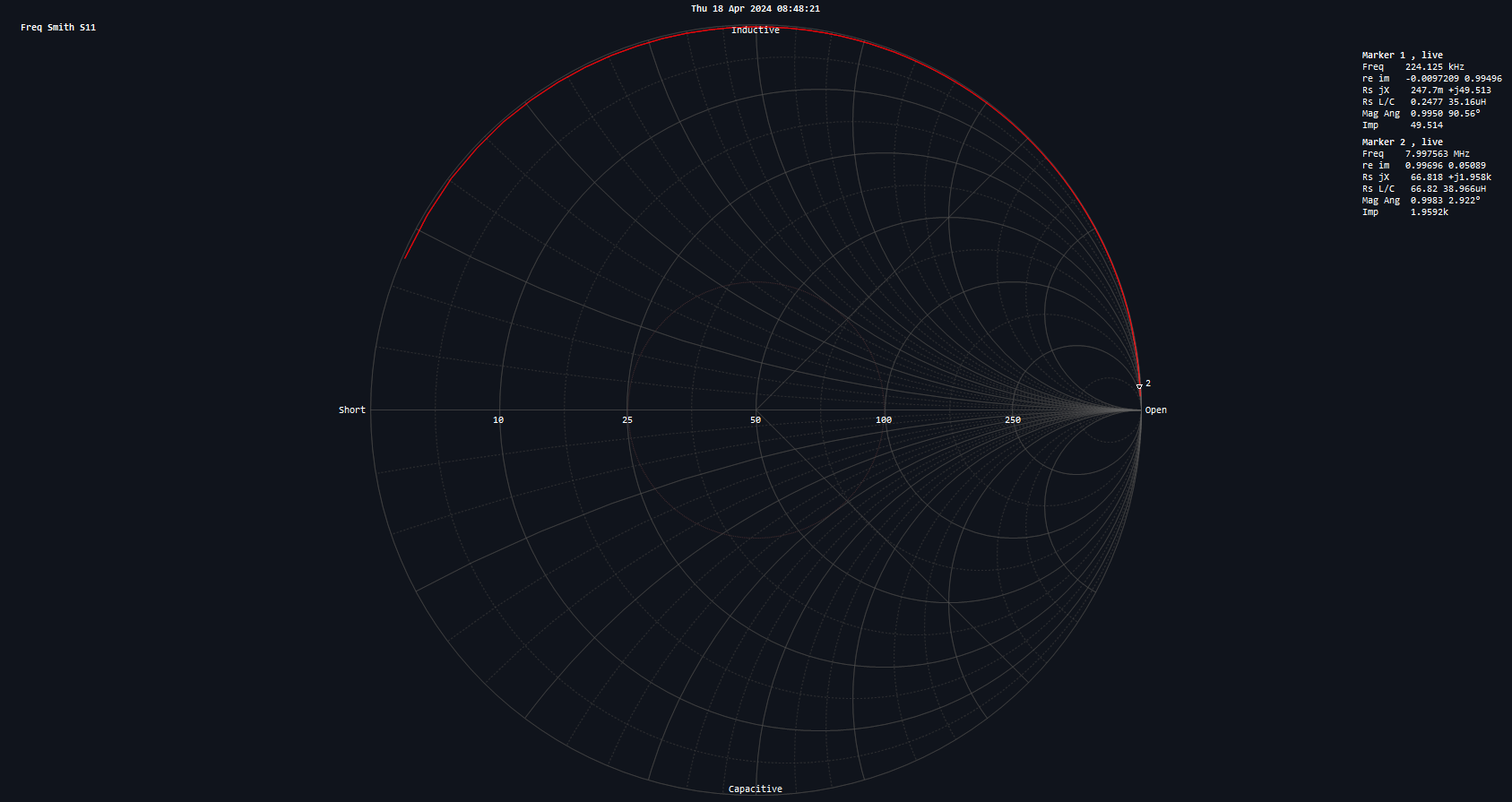
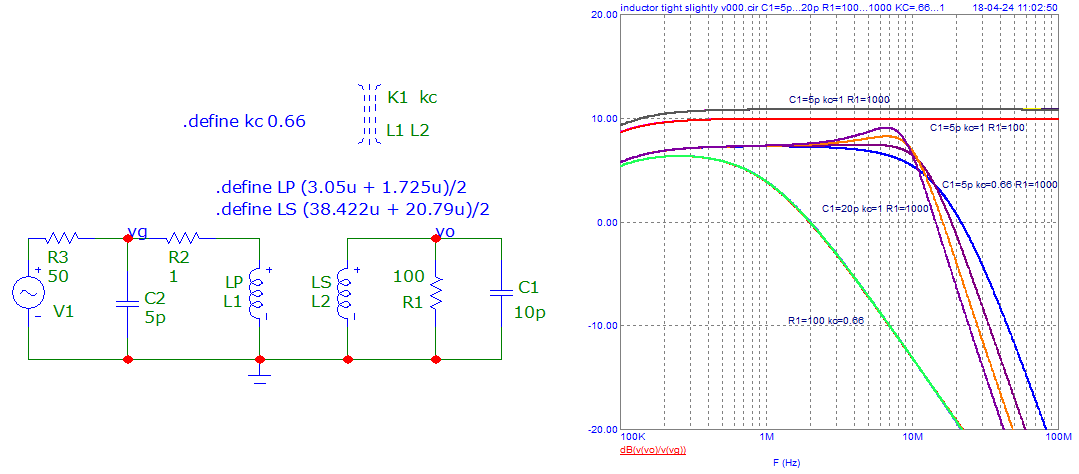
I have a transformer with a turns ratio of 6:24 (primary to secondary turns). I measured the primary inductance to be 3.05 microhenries (uH) with the secondary open and 1.725 uH with the secondary shorted. Similarly, the secondary inductance was 38.422 uH with the primary open and 20.79 uH with the primary shorted.
Using these measurements, I calculated the coupling coefficient (k) to be 0.66. This indicates that the magnetic flux is not perfectly coupled between the primary and secondary windings.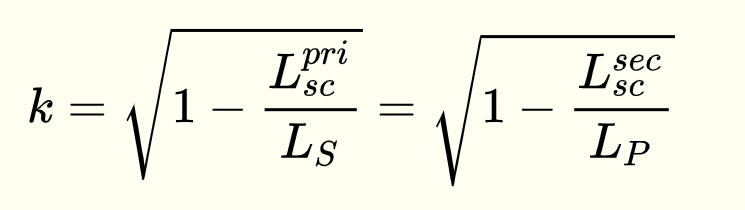
In an ideal transformer (k = 1), the voltage ratio would simply be the turns ratio (6/24). However, due to the imperfect coupling (k = 0.66), the actual voltage ratio will be slightly different.
I'm interested in understanding how the coupling coefficient affects the output voltage of the transformer and how does the coupling coefficient factor in while calculation the turns ratio.