How does a Current Injection clamp work from a physics persepective?

Question

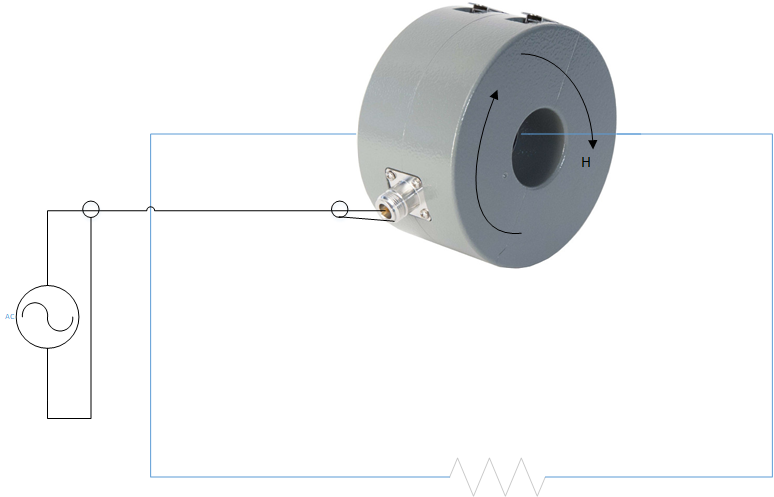
Current injection clamps are normally described as a transformer with a single turn secondary, which is all fine and good, but the construction of a transformer is different from a current clamp. Transformers are normally wound such that both the primary and secondary coils are wound around the same core. Seeing that the core is a usually a highly permeable material, nearly all the magnetic field produced by the primary flows through the core. This magnetic field then passes through the secondary coil which is tightly wound on the core and by Faraday's law produces a voltage.

A current clamp on the other hand has a coil on a core which is clamped around a wire. I understand that the wire forms a (sometimes very large) closed loop around the core, but it if the wire passes through the center opening of the core then the magnetic field travels through the secondary loop, but the magnetic field doesn't necessarily act on the wire itself (because the field is contained within the core material). So my questions are:

1. What is the physical mechanism that causes the voltage/current in the secondary. I understand that H field lines are crossing the circuit loops of the coil, but how physically is the current being produced? Is it the magnetic field acting on electrons and making them move? This doesn't seem to make sense to me.

2. Does a current clamp actually induce a current, or does it actually produce a voltage (like a transformer) which then develops a current based on ohm's law?

Answer 1

Although the majority of magnetic flux in a single turn transformer flows through the high permeability core, the so-called "leakage" flux is actually essential to transformer action.

The Poynting vector \$\vec{S}\$ describes the flow of power in an electromagnetic field and is given by

$$\vec{S}=\vec{E}\times\vec{H}$$

If the magnetic field intensity \$\vec{H}\$ were non-zero only within the core, then no power could be transferred via an electromagnetic field to or from a wire outside the core.

See Power Flow in Transformers via the Poynting Vector by Tapan Kumar Saha.

The non-zero flux outside of the core is commonly called "leakage" flux, but once it is understood that it is essential to transformer action, it's appellation as "leakage" seems less appropriate.

Now, to your post:

I understand that the wire forms a (sometimes very large) closed loop around the core, but it if the wire passes through the center opening of the core then the magnetic field travels through the secondary loop, but the magnetic field doesn't necessarily act on the wire itself (because the field is contained within the core material).

It is incorrect that the magnetic field doesn't necessarily act on the wire itself. It is incorrect that the (magnetic) field is contained (only) within the core material.

This latter idea, that a magnetic field can be totally confined within a toroid is true for an ideal static magnetic field, but not for a dynamic one. Extension of this idea to the dynamic case is a "lie told to children", that may have some value at some point in ones education (although I have my doubts), but which definitely hinders understanding at a later phase. It hinders understanding precisely in that it leads to confusion about how power is transferred to or from a wire passing through the center hole of a toroid.

Does a current clamp actually induce a current, or does it actually produce a voltage (like a transformer) which then develops a current based on ohm's law?

A changing magnetic field induces a Electro-Motive Force (EMF). This EMF causes current to flow through the wire (assuming it forms a closed circuit) according to Ohm's Law (if that is the governing relationship between voltage and current in that circuit).