Calculation of inductance

Question

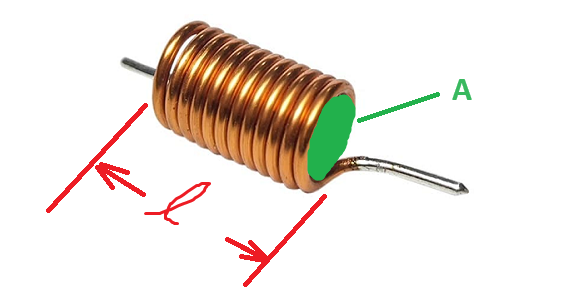
I know that inductance can be calculated with the formula $$ L = \frac{µN^2A}{l} $$ where \$L\$ is inductance, \$µ\$ is magnetic permeability(1 in air), \$A\$ is the area of the coil(cylinder flat face), \$N\$ is the number of turns and \$l\$ is the length of the inductor.

However, regarding length, is it utilised as the literal length from top to bottom of the inductor(e.g. the inductor is 2cm long), or is it used as the total unwound length of the inductor(e.g. 10cm of wire was used to make this inductor)?

Answer 1

\$µ\$ is magnetic permeability(1 in air)

Nope. \$\mu\$ is the product of the relative permeability of the core material (air/vacuum, ferrite, whatever), \$\mu_r\$, and the air/vacuum permeability, \$\mu_0\$:

$$ \mu=\mu_0 \ \mu_r $$

Air's magnetic permeability is \$\mu_0=4\pi \ 10^{-7}\$ Henries per metre, and its relative permeability is 1.

The correct formula is

$$ L = \mu_0 \ \mu_r \ \frac{A \ N^2}{\ell} $$

is it utilised as the literal length from top to bottom of the inductor(e.g. the inductor is 2cm long)

Yes, \$\ell\$ here is the effective (final) length of the coil.

^{Original image}

For transformers where magnetic circuits are involved, \$\ell\$ becomes the Magnetic Path Length.