0
\$\begingroup\$

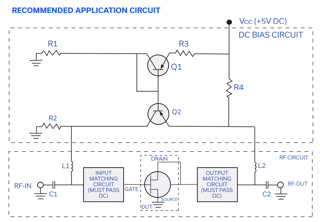
I am looking into the datasheet of a SAV-541+ E-PHEMT RF transistor, and looking at the recommended biasing circuit, im not sure how the drain current is determined.

Image from datasheet

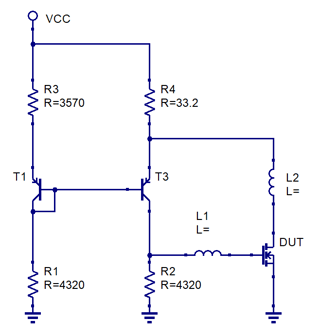
Above is the picture from the datasheet of the circuit. Below i have redrawn it, as i believe it makes the current mirror drawn more typical:

Biasing circuit redrawn

How do i calculate the drain current in this circuit, and more importantly, be able to calculate other resistor values for different current and drain voltages? It would make more sense to me, if the Drain-source of the device would be connected where R2 is. Of course this would leave the gate voltage unbiased.

For the values in the schematic, the drain current should be around 60 mA with a 3 V at the drain. VCC is 5 V. For this E-PHEMT device, \$V_{GS}\$ is around 0.5V for this drain current.

Also i wonder how tolerant this circuit is on varying gate threshold voltage \$V_{th}\$.

\$\endgroup\$
5
  • \$\begingroup\$ How mathy of an answer do you want? Closed solution? And can it be limited to the six parts on the left side of your redrawn schematic? \$\endgroup\$
    – periblepsis
    Commented Oct 16 at 20:35
  • \$\begingroup\$ @periblepsis, i was hoping for a simple solution with assumptions like 0.6V B-E voltage, etc. where it is possible to see how the gate voltage is set compared to the drain current. Now that i think more about the answer, i guess an approximate value for the DUT gate voltage is needed as well maybe? \$\endgroup\$
    – Linkyyy
    Commented Oct 17 at 14:47
  • \$\begingroup\$ It's not quite so simple to do from theory has there is a 10X change in collector currents for each 60 mV change in Vbe. So simplifying the B-E voltage is off the table (in my opinion.) So I'll leave this to others. \$\endgroup\$
    – periblepsis
    Commented Oct 17 at 21:51
  • \$\begingroup\$ @periblepsis, i was afraid it was not that simple, but thanks alot for your input. \$\endgroup\$
    – Linkyyy
    Commented Oct 18 at 15:04
  • \$\begingroup\$ You will never be able to understand a current mirror and/or its various incarnations such as the one you presented here without abandoning the over-simplified view. In fact, it's the kind of circuit that I would use to challenge and/or test someone claiming to understand important bipolar circuits. \$\endgroup\$
    – periblepsis
    Commented Oct 19 at 4:51

2 Answers 2

Reset to default
1
\$\begingroup\$

With VCC = 5V, Veb = 0.6V and resistor values as shown:

Id = [R3 * (VCC - Veb) / (R1 + R3)] / R4 = 59.96 mA

and

Vd = VCC - (Id * R4) = 3.01 V

\$\endgroup\$
3
  • \$\begingroup\$ it assumes all current through R4 is going through the DUT. I guess this this an alright approximation, but only when the current through R2 is << the drain current. \$\endgroup\$
    – Linkyyy
    Commented Oct 16 at 20:02
  • \$\begingroup\$ @Linkyyy - Of course a small part of what I labeled "Id", (<0.5% as a "guesstimate"), is flowing through T2/R2 to get the appropriate gate voltage. As Mini-Circuits only provides bare minimum specs in the datasheet, with focus on the RF side, there is no way to tell how much current that is. \$\endgroup\$
    – Raonoke
    Commented Oct 17 at 23:50
  • \$\begingroup\$ Thanks for your input! \$\endgroup\$
    – Linkyyy
    Commented Oct 18 at 15:07
1
\$\begingroup\$

You set the DC drain voltage according to the gain you want to achieve; see the last graph on datasheet page 4. You'd achieve variations be altering the R3/R4 ratio.

60 mA seems to be a sweet spot, however. I would only change these resistors were I to use a different supply voltage.

\$\endgroup\$
2
  • \$\begingroup\$ Thanks for the answer Marcus. I am aware that the rf performance can be altered by changing the drain current, but im more interested in how the drain current and voltage is calculated in the shown biasing method \$\endgroup\$
    – Linkyyy
    Commented Oct 15 at 19:48
  • 1
    \$\begingroup\$ I'll go with "with a circuit simulator"; this is a circuit with four resistors and two nonlinearities, so nothing you want to do by hand \$\endgroup\$
    – Marcus Müller
    Commented Oct 15 at 19:54

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.