Output LC filter transfer function

Question

I was trying to construct small signal analysis for phase shift full bridge converter. Below is the point that I reach.

According to the document, the transfer function of the output filter is given as in the 2'nd picture. I can derive it, it is easy. It is just a transfer function of LC filter with the Rload.

My question is as you can see in the 1'st picture, document calculated the input impedance and output impedance of the output filter.I didn't understand anything where these two equation come from. How can I derive it? and where does these two equation come from?

Secondly, what is this Δf? don't we generally equate the transfer function to the Vout/Vin?

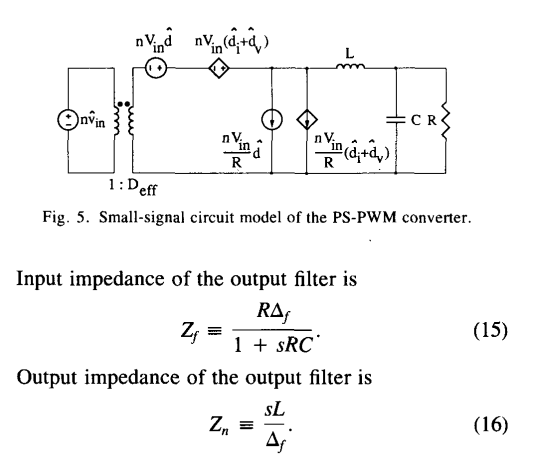Source: https://www.researchgate.net/publication/3279135_Small-signal_analysis_of_the_phase-shifted_PWM_converter/link/545ce4bc0cf2c1a63bfa58b2/download?_tp=eyJjb250ZXh0Ijp7InBhZ2UiOiJwdWJsaWNhdGlvbiIsInByZXZpb3VzUGFnZSI6bnVsbH19

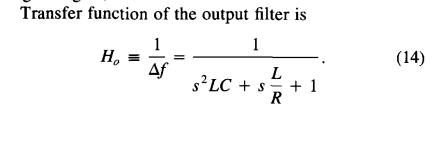Source: https://www.researchgate.net/publication/3279135_Small-signal_analysis_of_the_phase-shifted_PWM_converter/link/545ce4bc0cf2c1a63bfa58b2/download?_tp=eyJjb250ZXh0Ijp7InBhZ2UiOiJwdWJsaWNhdGlvbiIsInByZXZpb3VzUGFnZSI6bnVsbH19

Answer 1

How can I derive it ? and where does these two equation come from ?

The input impedance is simply \$sL+\dfrac{\frac{R}{sC}}{R+\frac{1}{sC}}\$

In other words it's the inductance in series with the parallel combination of R and C.

It reduces to this: -

$$Z_{IN} = \dfrac{s^2LC + s\frac{L}{R}+1}{1+sCR}\times R$$

And, as you can see from formula 14 in your image for \$\dfrac{1}{\Delta f}\$ the above equation becomes this: -

$$Z_{IN} = \dfrac{\Delta f\cdot R}{1 + sCR}$$

I'll leave it for you to calculate the output impedance but, as a clue, you can assume that the input to the filter comes from a voltage source (phase shifted PWM is theoretically a voltage source) so, you just short the input as you would when calculating a Thevenin impedance.

This means that R, L and C are in parallel and calculating that impedance shouldn't be too troublesome.

secondly, what is this Δf ? don't we generally equate the transfer function to the Vout/Vin ?

If you look at formula 14 you'll see that it is the transfer function. I have no idea why they also choose to call it \$\dfrac{1}{\Delta f}\$ but maybe a deeper read of your document will explain this.