VG1 is an AC (sine wave) signal with a voltage of ±50V. I need to scale down this voltage and shift it from bipolar (±3.3V) to unipolar (0 to +3.3V) using an op-amp. The op-amp should operate with supply voltages of 0 to +3.3V and the output should be 0 to +3.3V. kindly help me to do reverse calculation as well - Need to measure the VG1 voltage from op amp output through stm32.
1 Answer
If I understand the question correctly, what is desired is for +50 V input to result in 3.3 V output, and -50 V input to result in 0 V output.
If that is correct, the function that needs to be immplemented is
\$V_{out} = (0.033\times V_{in}) + 1.65\$
This can be accomplished completely with resistors and voltage reference.
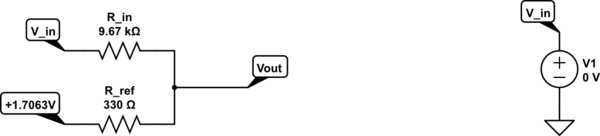
simulate this circuit – Schematic created using CircuitLab
We can calculated the needed resistors and reference voltage as follows.
Using Millman's theorem:
$$V_{out} = \frac{R_{in}V_{ref}+R_{ref}V_{in}}{R_{in}+R_{ref}}$$
therefore:
$$\frac{\Delta V_{out}}{\Delta V_{in}}= \frac{R_{ref}}{R_{ref}+R_{in}}$$
$$\frac{\Delta V_{in}}{\Delta V_{out}}= 1 + \frac{R_{in}}{R_{ref}}$$
$$\frac{100}{3.3}-1=\frac{R_{in}}{R_{ref}} = \frac{967}{33}$$
To calculate the needed reference voltage
$$V_{ref} = 1.65 \times \left(1+\frac{33}{967}\right) = 1.65\times\frac{1000}{967} \approx 1.7063$$
Now, it is unlikely that one has a 1.7063 V reference on hand, so it must be synthesized. We choose a 2.495 V TL431 and ground to synthesize 1.7063 and a 330 \$\Omega\$ resistance. Again, using Millman's theorem:
$$1.7063 = 2.495 \frac{R_{0}}{R_{0}+R_{2.495}}$$
$$R_{2.495} = 0.4622\times R_0$$
and using the parallel resistor formula
$$\frac{R_0\times R_{2.495}}{R_0+R_{2.495}}=330$$
\$R_0 = 1044 \;\Omega\$
\$R_{2.495} = 482.5 \;\Omega\$

The completed circuit, using a TL431 is given here:
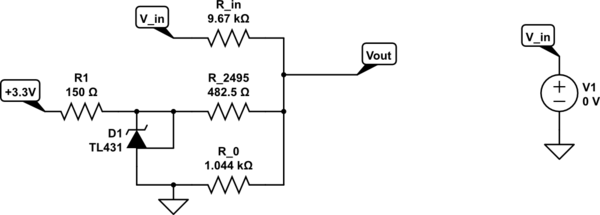
Which gives us the correct output (assuming we understood the question correctly).
Obviously, the resistance values may be scaled, and/or op-amps added to change impedances if necessary.
I made no attempt to find resistors that are found in common E-series. The values given in this answer may be synthesized from multiple resistors, and/or may be approximated if precision may be sacrificed to some degree.