Pulse-excited integrator reaching infinite potential?

Question

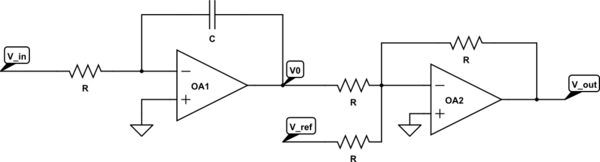
I was given the following Op-Amp circuit to analyse and to find the potential V_out as a function of time, with V_in defined as the Heaviside step function:

$$V_{\text{in}}=\begin{cases}0\mathrm{V} & t<0 \\ 1\mathrm{V} & t \geq0\end{cases}$$

^{simulate this circuit – Schematic created using CircuitLab}

I first begin by finding Vo as a function of V_in, by equating currents and using that the current going into the inverting input of OA1 must be 0A:

$$\frac{V_{\text{in}}}{R}=C\frac{\mathrm{d}V_{0}}{\mathrm{d}t}\implies V_{0}=\frac{1}{RC}\int_{-\infty}^{t'}V_{\text{in}}\:\mathrm{d}t$$

Then again at OA2:

$$\frac{V_{\text{out}}}{R}=\frac{V_0}{R}+\frac{V_{\text{ref}}}{R} \implies V_{\text{out}}=V_0+V_{\text{ref}}$$

Combining the two equations gives:

$$V_\text{out}=\frac{1}{RC}\int_{-\infty}^{t'}V_{\text{in}}\:\mathrm{d}t+V_{\text{ref}}$$

When we use V_in as defined above and with R = 100kΩ, C = 10µF and V_ref = 10V, we get:

$$V_{\text{out}}=\begin{cases}(t+10)\mathrm{V} & t\geq0 \\ 10\mathrm{V} & t<0\end{cases}$$

However, this means that we end up with:

$$\lim_{t\to\infty}V_{\text{out}}=\infty\mathrm{V}$$

Which suggests that I've done something very wrong here? Or is this just a consequence of using the ideal Op-Amp assumption?

Answer 1

You haven't done anything wrong. The first stage of your circuit is an integrator, and when you integrate a step function (your input) you get a ramp. Since your input never ends, the ramp never ends and the final value is infinity.

Of course, this only holds for an ideal op-amp. In a real op-amp, output voltages would be limited to the rail voltage.

In case you're wondering, I've never seen the output voltage limitations accounted for in equations. You just have to introduce a nonlinear element into the model (a voltage limiter) and then all of your linear equations are essentially out the window. In other words, the linear equations you're used to are all well and good as long as the output of any of your stages doesn't exceed the rail voltage - as it will in this case.