I need help with the following problem:

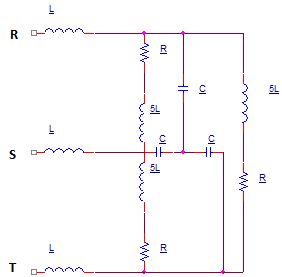
Given symmetric three phase system (see attachment) of phase voltages with angular frequency ω=100rad/s, R=5ωL=100Ω. Find capacitance of capacitor C such that the power factor of three phase receiver has maximum value.

After transformation of Y capacitors to Δ (see attachment), it gives $$C_1=C/3.$$

Now we have a Δ connection of impedance Z which is a parallel of R,j5ωL and C1. Let $$\underline{Z_1}=R+j5\omega L.$$ From given data we can find that L=0.2H. This gives $$\underline{Z_1}=100(1+j)\Omega.$$ Now

$$\underline{Z}=\frac{\underline{Z_1}\cdot(-jX_{C_1})}{\underline{Z_1}+(-jX_{C_1})}=\frac{300(3+j(3−2⋅10^4C))}{2⋅10^8C^2−6⋅10^4C+9}\Omega $$

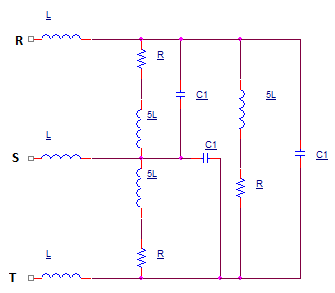
Now we have a three phase system with receiver in Δ connection (see attachment):

After Δ to Y transformation (see attachment), we get new impedance: $$\underline{Z_2}=\frac{\underline{Z}}{3}=\frac{100(3+j(3−2⋅10^4C))}{2⋅10^8C^2−6⋅10^4C+9}\Omega.$$

Let

$$\underline{Z_3}=\underline{Z_2}+jX_L=\underline{Z_2}+j20=\frac{20(15+j8(3−2⋅10^4C+25⋅10^6C^2))}{2⋅10^8C^2−6⋅10^4C+9}\Omega.$$ Now we have a clean Y receiver connection with impedance Z3 (see attachment):

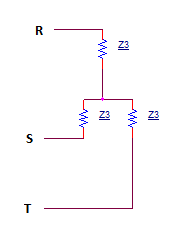
Question: We are not given any values for voltage, current or power, so how to express power factor cosϕ without knowing any of those values?