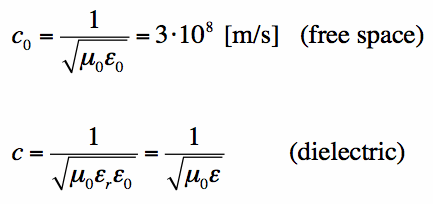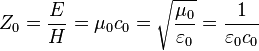The velocity that electricity propagates at is related to the dielectric in the cable. In free-space, an EM wave travels at the speed of light but there is only the permittivity of free space (\$\epsilon_0\$)to hinder it: -
So, as the permittivity (\$\epsilon_r\$) rises, velocity decreases. Magnetic permeability is the other factor but this barely changes between a cable and free-space.
The characterisitic impedance of free space is also related to those terms: -
Basically, if you compared both equations, as characteristic impedance drops (due to permittivity increasing), velocity of propagation also drops.
The product of speed and impedance is \$\dfrac{1}{\epsilon}\$
And the ratio of impedance to speed is \$\mu_0\$.
I've used \$\mu_0\$ above (rather than \$\mu\$) because there is no significant increase in magnetic permeability due to a cable's construction.