When I see the amount of lines thrown on the blank page by the OP as an attempt to derive this 3rd-order transfer function, I think it is about time time that fast analytical circuits techniques or FACTs are taught in universities. The principle is simple and, very often, works with inspection: just look at the circuit to infer the time constants without writing a single line of algebra. The exercise consists of determining the resistance \$R\$ offered by each energy-storing element when the circuit is observed with a zeroed excitation or with a nulled response. Once you have the \$R\$, you can form a time constant expressed as \$\tau=RC\$ or \$\tau=\frac{L}{R}\$.
So we start with a bunch of small sketches to determine the natural time constants of this linear circuit. We reduce the excitation to zero volt (the input source is replaced by a short circuit) and you "look" through the energy-storing element temporarily disconnected from the circuit to find the resistance \$R\$ driving that element:
As you can see, you just inspect the drawing to see, in your head, what resistance the terminals in question offer (where the arrow points). And if you make a mistake, it is easy and fast to come back to the circuit and correct the resistance \$R\$ you mistakenly determined.
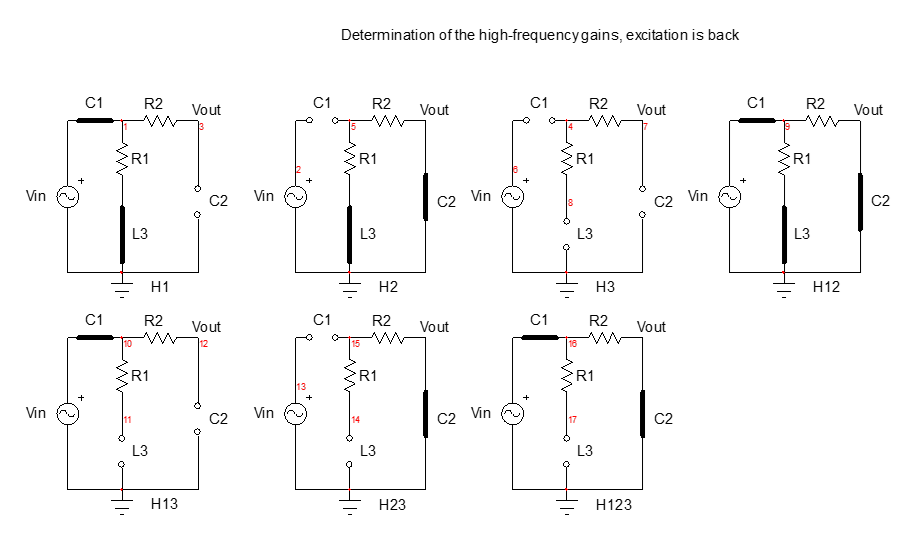
For the zeroes, you can do what is a called a null double injection (NDI) but I often prefer for the passive case, to determine high-frequency gains: the source is back in place and you inspect the circuit to determine what the gains are when energy-storing elements are alternately placed in their high-frequency state:
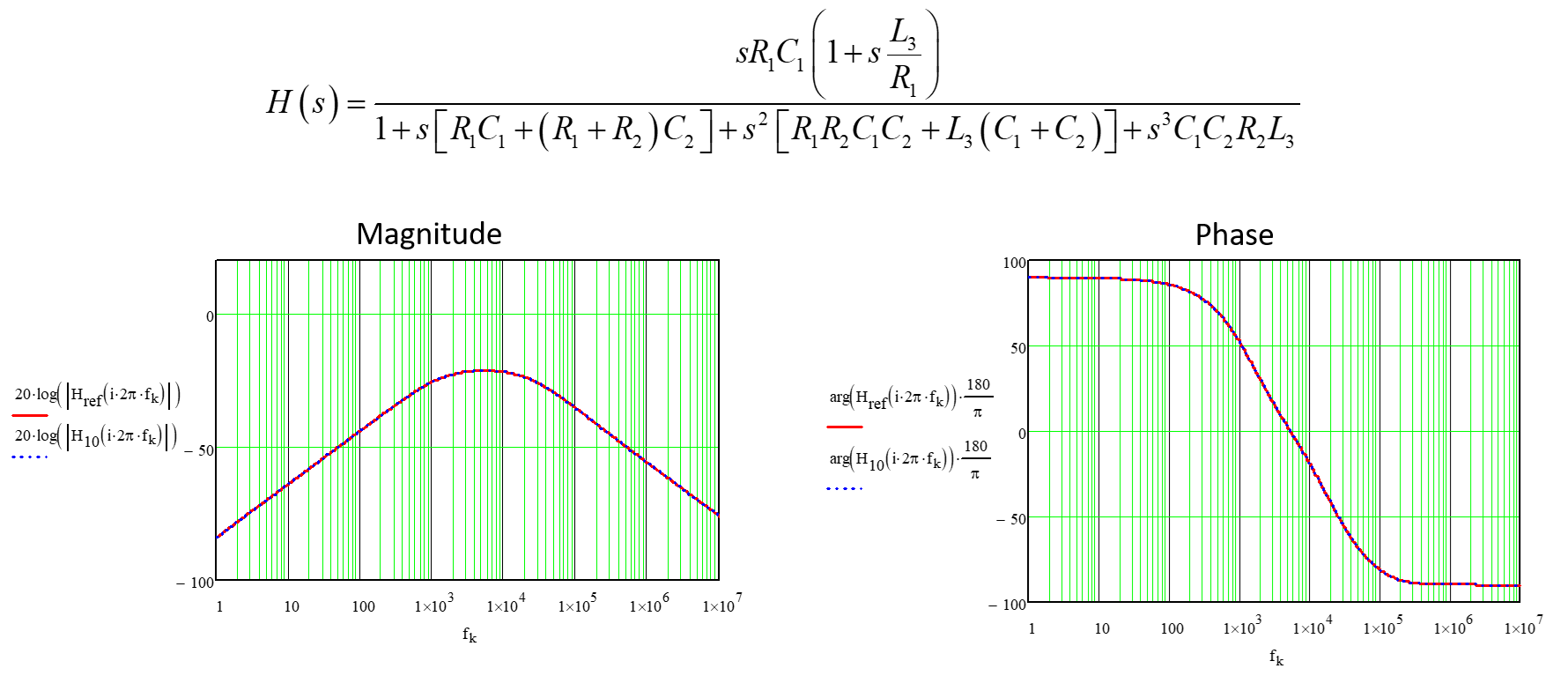
You can see how easy it is! When you have all these elements on hand, you assemble them in a Mathcad sheet which a) will check homogeneity of the formulas and b) will let you compare the response obtained by the FACTs and that delivered by the brute-force approach (Thévenin in this case):
Then you can plot the magnitude and phase while comparing the two responses in similar plots. They perfectly match as shown below:
You can see how easy the FACTs are compared to the classical KVL and KCL methods. You apply the divide-and-conquer strategy promoted by Dr. Middlebrook and solve your circuit step-by-step with the ability to come back and solve an intermediate wrong result. There is no way you can do that with the brute-force approach.
Acquiring the FACTs skill is simple and you can start with 1st-order circuits as shown in the APEC seminar I taught in 2016. Then, for an in-depth description of the method up to the order \$N\$, you can have a look at the book I published on the subject.