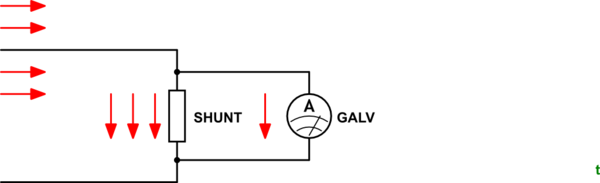
Let's look at a very simple example first. We have a galvanometer that has a full-scale reading of \$ I_G \$ and we want to measure currents up to four times that value.
<!-- Begin schematic: In order to preserve an editable schematic, please
don't edit this section directly.
Click the "edit" link below the image in the preview instead. -->
<!-- End schematic -->
*Figure 1. By making \$ R_S = \frac {1}{3}R_G \$ three quarters of the current will be handled by the shunt and the remaining quarter by the galvanometer.*
Note that whatever current is flowing in the circuit being measured that the current will always split 3:1 due to the ratios of the shunt and galvanometer resistances.
If we need to measure currents of 100 times the galvanometer's max rating then we need a shunt of \$ R_S = \frac {1}{99}R_G \$ to divert 99% of the current from the galvanometer.
>I understand why the shunt resistance has to be of a smaller value and the resistance of voltmeter has to be of a really greater value but what I fail to comprehend is how can we successfully measure current when the deflection of the needle is going to always be maximum due to the passage of Ig current
Remember that the galvanometer doesn't "hog" all the current if the current is a low value. The current always splits inversely to the ratio of the resistances.