In the circuit below what additions are needed so that charge will move from Ch to Ca, given that the charge on Ca can be higher that Ch?
In this system (see image below, it's a thought experiment for me at this stage), it is possible for the capacitance of the capacitor to dynamically change.
ps. this question is a simplification of an argument that occurred between two electrically inexperienced people, me being one of them.
Thanks
Charge will move from a higher potential (voltage) to a lower potential. In the fluid analogy, fluid current moves from a high pressure to a lower pressure. In the same fluid analogy, charge is equivalent to a volume of fluid and a small volume of fluid can be at a higher pressure than a larger volume.
In electrical terms, Q = CV, where Q is charge, C is capacitance and V is voltage. So a small capacitor charged to a high voltage could hold less charge than a high value capacitor charged to a lower voltage. Connecting the two together would result in a current flowing from the small capacitor (with less charge) to the large capacitor (with more charge).
As to whether the capacitance can change dynamically, well yes if the area of the capacitor's plates or the distance between the plates changes or potentially, (no pun intended) if the dielectric constant of the material between the plates changes.
In order to move charge from a capacitor with lower voltage to another capacitor with higher voltage, you will need to add an energy source to the circuit. This is a passive circuit and the voltage will only tend towards equilibrium.
If the capacitance of the caps can change, then you can do it without an actual voltage or current source. You'd need to change the capacitance of the source in the direction that will cause its voltage to increase, or vice versa for the other cap.
q = C⋅V, so to increase voltage, you need to decrease capacitance. So if you separate the plates of Ch enough, it should increase the voltage of Ch enough to drive charge into Ca. Note that you're still using an energy source, but it's mechanical now instead of electrical.
(This is actually the reason why people thought capacitors stored charge on the surface of the dielectric instead of on the plates. When they charge it and disassemble the plates, the voltage rises enough that the air breaks down and the charge gets deposited onto the dielectric.)
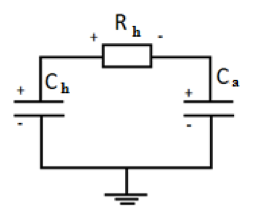
If by "charge" you mean the voltage over the capacitor it's impossible for current to flow from \$C_H\$ to \$C_A\$ if the voltage over the latter is higher. If by "charge" you mean energy it's different.
The energy in a capacitor is \$\dfrac{V^2 C}{2}\$. If energy flows from the capacitor at the highest voltage to the other one energy is lost in the resistor. Suppose both capacitors are equal capacitance, \$C_A\$ being at \$V\$ volt, the other one at zero volt. Then the total energy of the system is \$E = \dfrac{V^2 C}{2} + 0\$ before current starts to flow. After both capacitors are connected through the resistor current starts to flow until there's an equilibrium, where the voltage on both capacitors is \$\dfrac{V}{2}\$ volt. The energy is then \$E = \dfrac{\left(\dfrac{V}{2}\right)^2 C}{2} + \dfrac{\left(\dfrac{V}{2}\right)^2 C}{2} = \dfrac{V^2 C}{8} + \dfrac{V^2 C}{8} = \dfrac{V^2 C}{4}\$. That's half of the energy we started with! Where has the other half gone? That's dissipated in the resistor as heat.
If you would eliminate the resistor and connect the capacitors directly, you would still lose half of the energy you started with, but then most of that energy will be radiated as RF energy in the spark you would get during shorting.
Translating to the hydraulic model you can see the voltage over the capacitor as a water level (water pressure is also used, but a higher water column gives a higher pressure, so that's OK). The capacitance is then the water capacity of the tank. If you would connect a full tank to an empty tank of the same capacity, both tanks will end half full, i.e. their water levels halved. Which agrees to half the voltage from the calculation.
