I was learning to work with RLC circuits with sinusoidal excitations and the book I was dealing with showed me that the responses of these passive elements to sinusoidal excitation were themselves purely sinusoidal and hence phasor digaramsdiagrams were the best way to deal with these elements, but then.
When I tried solving a simple series RLC circuit using laplaceLaplace/complex frequency analysis and, I landed myself a sine term, a cosine term, and a real exponential term.
I don't know what or why I am getting that real exponential term,. I tried to do it over and over and I got the same results. I am pretty sure my calculations are correct but the book mentions nothing about the exponential term. Kindly pitch in...
This is the circuit I was talking about:
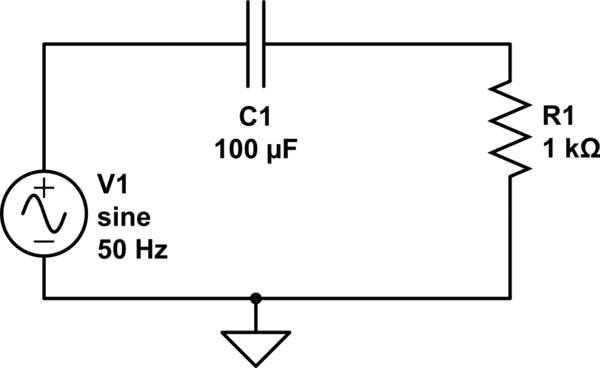
simulate this circuit – Schematic created using CircuitLab
Here is an image for a KVL and laplaceLaplace transformation:
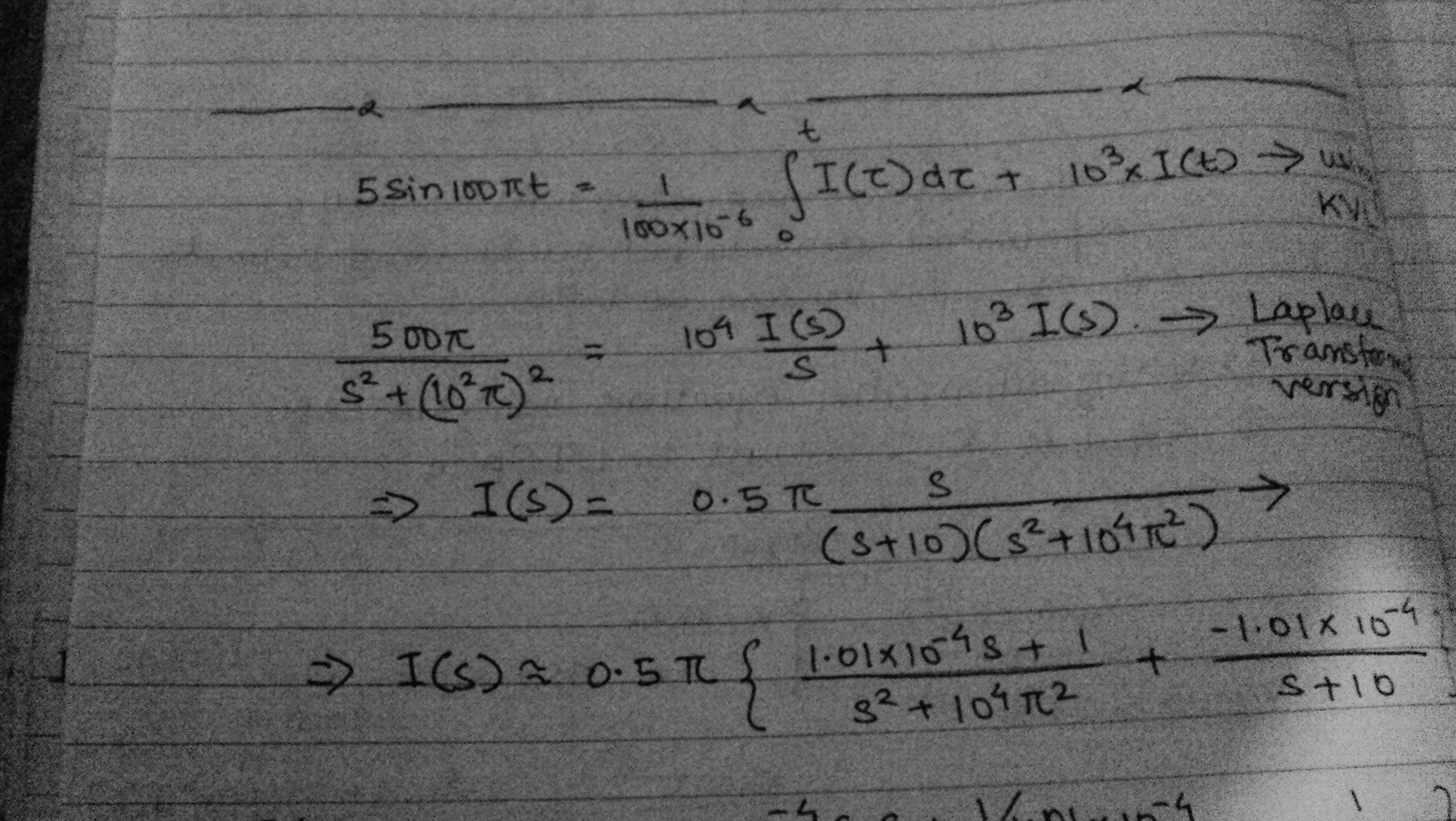
Here is an image for the inverse after separation. I dontdon't think there is a mistake.

