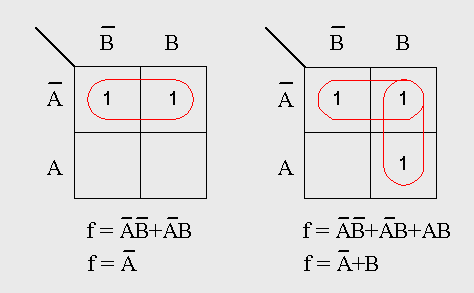
If your book is any good, it should mention Karnaugh mapping. Karnaugh mapping is about grouping like values (like all ones or all zeros) on a map consisting of rows and columns of logical combinations.

If you can group logical combinations like that you can simplify your logical function as given in this example.
Another method which works better for me is to work with truth tables. On the left you write all logical combinations of the inputs. For 3 inputs that would be
A B C
0 0 0
0 0 1
0 1 0
0 1 1
1 0 0
1 0 1
1 1 0
1 1 1
Note that this table becomes rather long if you have many inputs: \$2^N\$ lines. On the right you write the output(s). For example
A B C Y
0 0 0 1 0
0 0 1 0 1
0 1 0 1
0 1 1 0
1 0 0 0 1
1 0 1 1 0
1 1 0 0
1 1 1 1
With some exercise you often can see a pattern in the output. In this case for the first four lines, where A = 0, Y = B XOR C. For the rest, where A = 1, Y = NOT (B XOR C), or, combined: Y = A XOR B XOR C. (This can be used to create a parity bit)
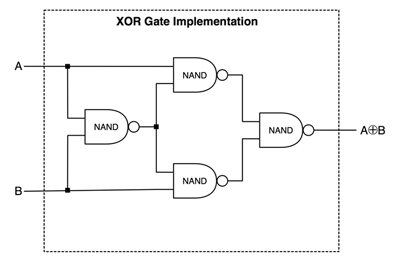
Jeff mentions how you can use DeMorgan's Law to create an OR gate from NANDs. This XOR gate is also basic: