The impedance of a capacitor is given by the formula:
$$Z_C = \frac 1 {j \omega C} = \frac 1 {j 2 \pi f C}$$
where \$j = \sqrt{-1}\$. It takes a bit of algebra to get the negative sign:
$$\frac 1 j = \frac j j \cdot \frac 1 j = \frac j {j^2} = \frac j {-1} = -j$$
$$Z_C = \frac 1 j \cdot \frac 1 {\omega C} = \frac {-j} {\omega C}$$
The reactance is the imaginary part of the impedance, so you could say that it's:
$$X_C = Im\{Z_C\} = -\frac {1} {\omega C}$$
If you want to combine series inductors and capacitors into a single equivalent reactance, the sign matters.
But what the \$-j\$ really represents is a -90 degree phase shift between the capacitor's voltage and current (current leads voltage):
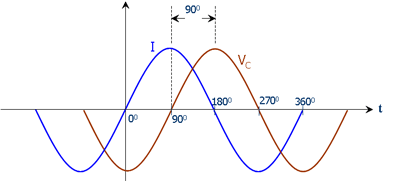 (source)
(source)
If you want to talk about the magnitude and phase shift effects of the reactance separately, then you can drop the negative sign:
$$Z_C = \frac 1 {\omega C} \angle -90^\circ$$ $$X_C = |Z_C| = \frac 1 {\omega C}$$
I wouldn't say either of them is wrong. They're different ways of simplifying to avoid complex numbers. Any simplification will be right at some times and wrong at other times. You need complex numbers to get the full picture, but that's a lot of math for a college freshman or the general public. So introductory books often deal with magnitude and phase effects separately.
Your citations are good examples of this. The first book gives the positive reactance but then tells you to combine inductance and capacitance like this:
$$Resultant\ reactance = X_L - X_C = 2 \pi f L - \frac 1 {2 \pi f C}$$$$\text{Resultant reactance} = X_L - X_C = 2 \pi f L - \frac 1 {2 \pi f C}$$
The second book gives the positive formula and describes phase shifts in the next paragraph. The third book (Electronics for Dummies) is a deliberate simplification. The fourth book describes the phase shift in terms of phasor diagrams on the next page. The fifth book mentions phase shifts in the box below the definition, but says that the book omits inductors entirely. The sixth book describes phase shifts on the page after the definition.
