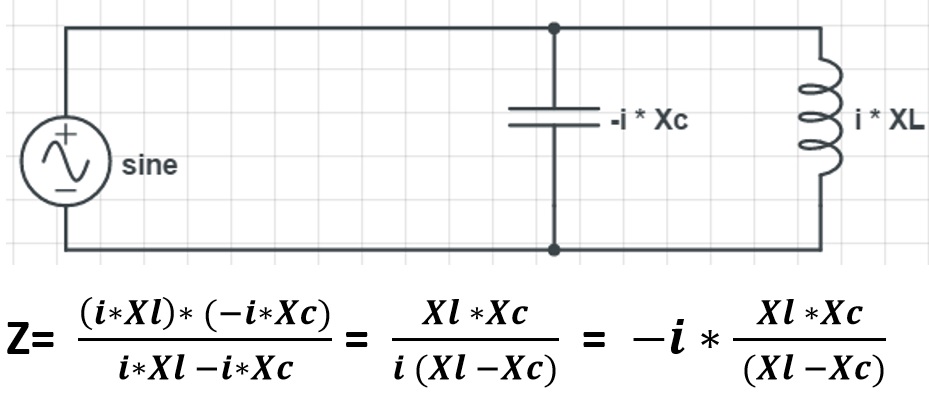In phasor or vector diagram, a capacitor that is parallel to the supply can improve power factor. I know this is practically true but I don't understand the mathematical equation:
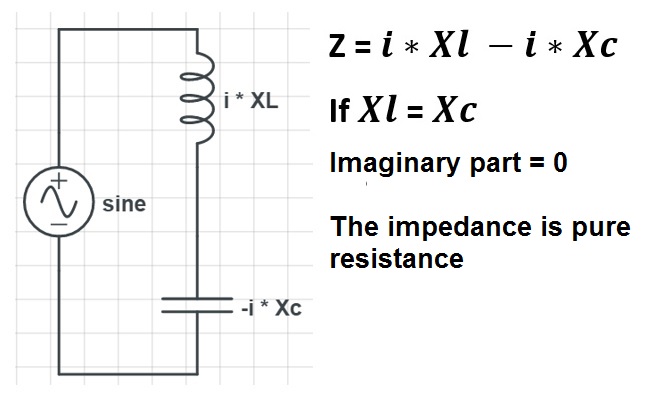
The total impedance (Z) of the following circuit has imaginary part i=root(-1). That means it has a reactants and it will consume reactive power.
If XL = Xc , the reacance should be infinity or has a very large value so it will consume large reactive power.
I feel like algebra does not support phasor diagram... Would you tell me what I'm missing?
Edit:
Here is the opposite case:
If a very high reactance is good for power factor, Here is a circuit with a very low reactance. Does it also improve power factor? If yes, which one is better?