To get 5V peak from a 10V peak supply across \$1 \mu F\$ capacitor at 100Hz we need current equal to
I = 5V/Xc = 5V/1.592kOhm = 3.1407mA and the voltage drop across resistor is
$$V_R = \sqrt{(10V^2 - 5V^2)} = 8.66V peak $$
therefore
$$R = \frac{8.66V}{3.1407mA} =2.757k\Omega $$
So yes, your calculations are correct.
And the simulation result look like this
[![enter image description here][1]][1]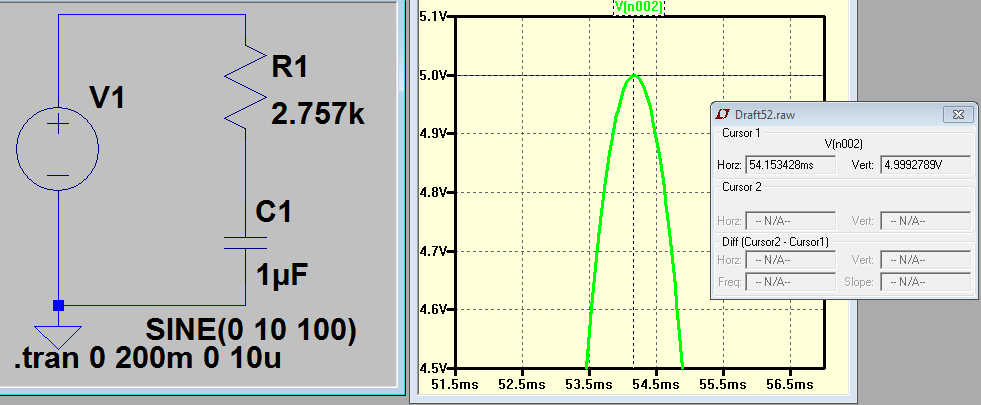
As you can see the peak voltage is 5V but RMS value is around 3.5V [1]: https://i.sstatic.net/6azUQ.png
