Alternator Simulation Completely revised answer...
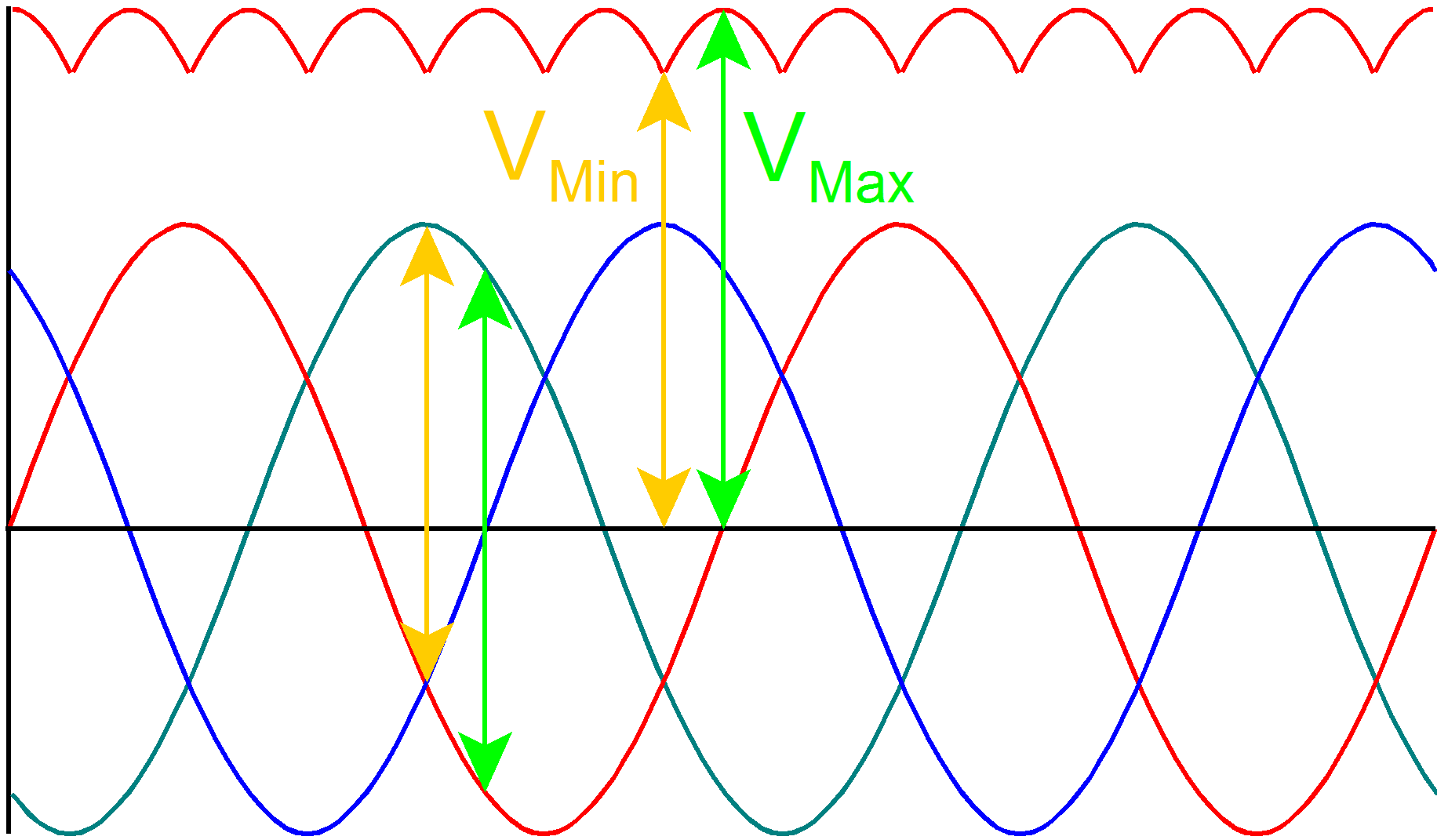
If the alternator produces 9V peak sine waves. Your Red Dot to Green Dot voltage will vary from -9V to 9V.
\$V_{Red} = 9 sin (\omega t) V \$
\$V_{Green} = 9 sin (\omega t\ -\ 120^{\circ}) V \$
\$V_{Blue} = 9 sin (\omega t\ +\ 120^{\circ}) V \$
$$V_{Min} = 9 sin (90^{\circ}) - 9 sin (90^{\circ} +\ 120^{\circ}) - 2 \times 0.7V = 12.1V$$
$$V_{Max} = 9 sin (120^{\circ}) - 9 sin (120^{\circ} +\ 120^{\circ}) - 2 \times 0.7V = 14.19V$$
The actual voltage seen by the battery will be line-to-line voltage across two phases minus the diode voltages.
We can determine this by looking at two points. Between \$60^{\circ}\$ and \$120^{\circ}\$, we can assume diodes associated with red and blue are conducting\$V_{Line} = \sqrt {3}\ V_{Phase} = \sqrt {3} \times 9V = 15.59V\$.
So at \$90^{\circ} \$, red and blue are conducting. Assuming 0.7V for diodes\$V_{Bat} = V_{Line} - V_{diodes} = 15.59V - 1.4V = 14.19V\$.
$$V_{Min} = V_{Red} - V_{Blue} - 2 V_{Diodes}$$ $$V_{Min} = 9 sin (90^{\circ}) - 9 sin (90^{\circ} +\ 120^{\circ}) - 2 \times 0.7V$$ $$V_{Min} = 9V - (-4.5V) - 2 \times 0.7V = 12.1V$$
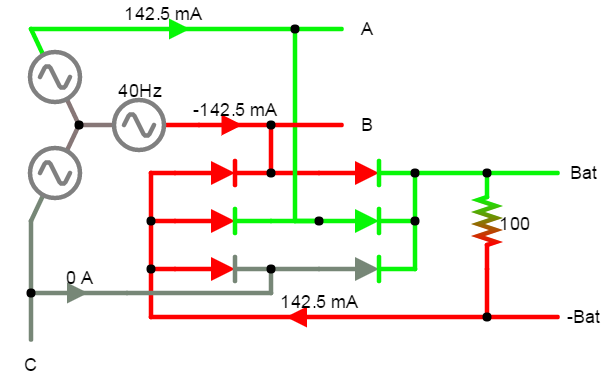
At \$120^{\circ}\$, red and blue are conducting. Green is 0\$I_{Bat} = \frac {V_{Bat}} {R} = \frac {14.19V} {100\Omega} = 141.9mA\$.
$$V_{Max} = V_{Red} - V_{Blue} - 2 V_{Diodes}$$ Maximum Power at Resistor: $$V_{Max} = 9 sin (120^{\circ}) - 9 sin (120^{\circ} +\ 120^{\circ}) - 2 \times 0.7V$$$$ P = \frac {V_{Bat}^2}{R} = \frac {(14.19V)^2}{100\Omega} = 2.01W $$ $$V_{Max} = 7.79V - (-7.79V) - 2 \times 0.7V = 14.18V$$
WhichThree-phase Power: $$ P = \sqrt {3}\ V_{Bat}\ I_{Line}\ cos \theta = \sqrt {3} \times 14.19V \times 141.9mA \times 1 = 3.487W$$ The Three-phase Power calculation is \$V_{Line} = \sqrt {3}\ V_{Phase} = \sqrt {3} \times 9V = 15.59V\$, minuswrong because of the 1.4V foraction of the diodes.
So depending
Only two sets are on at the maximum and minimum you see acrosssame time as shown by the battery terminal and blue dot, you shoulddrawing. This also means there will be able to work backwards to derive instantaneous waveforms for alternatorcurrent flowing in the neutral. Your red and green dots
Single-phase Power with Line voltages:
$$ P = V_{Bat}\ I_{Line}\ cos \theta = 14.19V \times 141.9mA \times 1 = 2.01W$$
This agrees with the load calculation.
At \$90^{\circ}\$Single-phase Power with Phase voltages: $$ P = \sqrt {3}\ V_{Phase}\ I_{Phase}\ cos \theta = \sqrt {3} \times (9V - 0.7V) \times 141.9mA \times 1 = 2.04W$$ Again, \$V_{Blue} = 50\% \ of \ V_{Red}\$ or atthis agrees. Because of the \$120^{\circ}\$\$\sqrt {3} \$, \$V_{Blue} = V_{Red}\$I'd go with line voltages.
Actual voltage will also depend on speed of rotationThe action of alternatorthe diodes also means the Green and Blue dots will NOT be at the same potential.
It's a Image shows +Bat varying from 8.35V to 3.86V, while -phase alternatorBat varies from -3.86V to -8.35V. This brings us back to the \$V_{Min}\$ calculation.
$$P_T = \sqrt {3} V_{Line} I_{Line} cos \theta $$
$$P_T = 3 V_{Phase} I_{Phase} cos \theta $$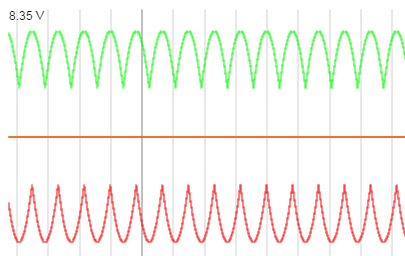
Turned into a dc battery charging circuit.$$V_{Min} = 8.35V - (-3.86V) = 12.21V $$
$$P_T = V_{Alternator} I_{Line}$$$$V_{Min} = 9 sin (90^{\circ}) - 9 sin (90^{\circ} +\ 120^{\circ}) - 2 \times 0.7V = 12.1V$$
TheYour Green and Blue dots shouldDot will be at 0V and the same potentialBlue Dot to Green Dot voltage will vary from -3.86V to -8.35V.
Then measure ALL 3 phase voltages at the same time. If they are all the same, then the Green and Blue dots are at the same potential.Minimum Power: $$ P = \frac {V_{Bat}^2}{R} = \frac {(12.1V)^2}{100\Omega} = 1.46W $$
You say they are NOT, but you provide no evidence. No measurements. No info on alternator. You say ignore this. It is not important. This (should be) a 3\$ \phi\$ alternator connected in wye with two diodes. It is fairly simple. The math is correct and the simulation gives close results. We cannot see what you see and you give us nothingNumbers should scale to work withreal world.
You have not been forthcoming with information, so this is my last on this. Have a nice day....To calculate phase voltage:
- Measure \$V_{Bat_{Min}}\$.
- Calculate \$V_{Phase} = (V_{Bat_{Min}}\ +\ 1.4V) \times \frac {2}{3}\$.
To calculate power (Max or Min):
- Measure \$V_{Bat_{Min}}\$.
- Measure \$I_{Bat_{Min}}\$.
- \$P = V_{Bat_{Min}} \ I_{Bat_{Min}}\$
- Repeat using Max quantities.