That 90º lag comes from the derivative with respect to time in the Faraday's law of induction:
(Differential form)
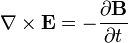
(Integral form)
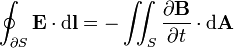
The left hand side of this last equation is the EMF. If those equations didn't have a derivate with respect to time, |EMF(t)| would be maximum when the apparent |B(t)| iswas maximum, but the (negative) derivative causes the sin(t) that there would be in EMF(t) to turn into a -cos(t), and that is where the 90º lag comes from.
