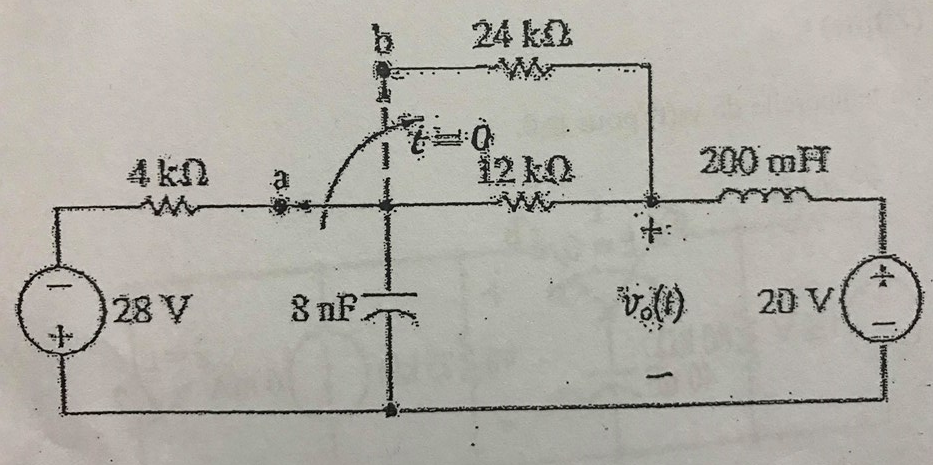
The switch in the following circuit was in position 'a' for long time , before passing to position b at t=0.
Find the initial current.
Find the initial Voltages of the capacitor , the inductor and the resistors.
What i tried
At t=0- , the circuit will look like this :
Since the switch was closed for a longtime , the current going through the capacitor will become 0 , while the voltage of the inductor will become 0.
Applying KVL : 28 +14I +20=0 , Hence I = -3A = I0
The voltage through the capacitor will be equal to the voltage through the 4K resistor + 28v
vc(t=0) = 4(-(-3))-28=-16V
and finally VL(t=0) = 0 since the inductor is short circuited.
However when the switch passes to the position b , the KVL equation doesn't apply anymmoreanymore: -16+8(-3)+0+20 is different than zero.
Thanks in advance.