Rev A.
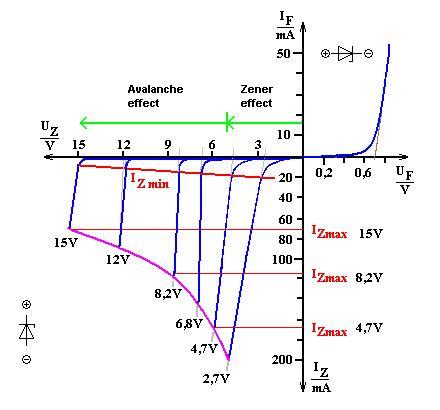
Symbol used is a Schottky Power Diode, which are Avalanche type clamps but like Zeners use the same notation for current, \$i_Z\$
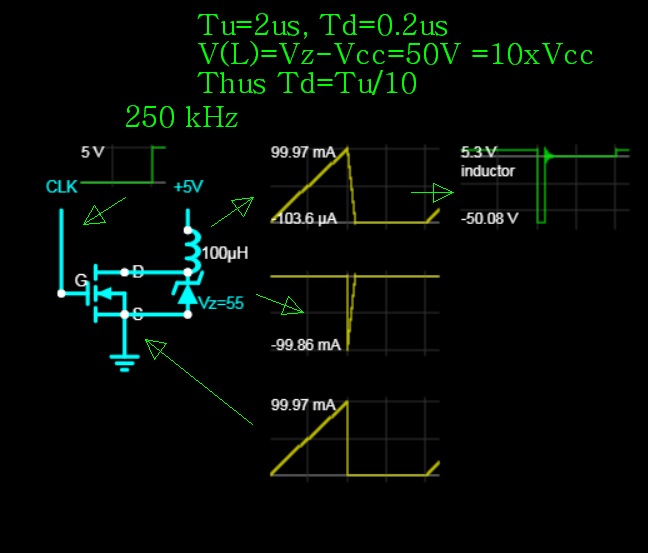
Although the energy stored in current in the Inductor is released into the Zener E= ½ LI² , I(t) is a ramp and not constant.
But what is the Power Dissipation?
Yet we know when the transistor is switched off, Iz=IL and for now, if we let Vce(sat)=0, the voltage across the inductor = 5V , thus the final power input while the ramp reaches a peak current , Ip is P = ½ VIp ( triangle is ½ the area of the VI product square)
We already concluded Vdt = LIp = some fixed value.
Although the semiconductor doping of Avalanche diodes is lighter, has a higher breakdown voltage, is faster and has a different conduction mechanism, the notation for such diodes often still uses \$i_Z\$.