From Prime Implicants and Essential Prime Implicants in K Map
S is an implicant of the function f if and only if the value S is equal to 1 so that the value of the function f is also equal to 1.
a minterm will be an implicant, but an implicant need not be a minterm.
So Digital Logic | Implicants in K-Map use of implicant is implying a minterm is an implicant, which disagrees with the above definition.
\$ ABC\$, \$ ABC \bar D\$ & \$ ABCD\$ are all implicants, but only 1 is a prime implicant.
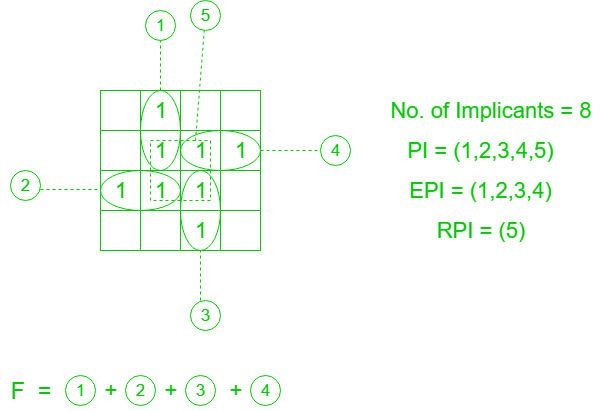
So 8 minterms + 5 prime = 13 implicants, 5 prime, 4 essential & 1 redundant.