For a constant load, how does increasing supply voltage increase speed of the motor?

• Speed ∝ voltage.
• Torque ∝ current.

Let's imagine the following:

• The motor is connected to the voltage source and is running at a constant speed. The back EMF has risen and the current has reduced from its starting value and is stable.
• If we now increase the voltage the current will increase. Remember that the current, \$ I = \frac {V_S - V_{bEMF}}{R} \$ where R is the motor resistance.
• The increased current will give increased torque which will accelerate the motor to its new steady speed.

But we know that speed is inversely proportional to armature current.

No. Speed is proportional to voltage. If you try to stall a motor running on constant voltage you will see the current rise. However, given a constant voltage the current will decrease as you approach the speed for that voltage. That may be the source of your confusion.

You could get a good feel for this if you have access to a lab power-supply with a voltage and current meter along with a suitable DC motor. Try running the motor in constant voltage mode an in constant current mode.

For a constant load, how does increasing supply voltage increase speed of the motor?

• Speed ∝ voltage.
• Torque ∝ current.

Let's imagine the following:

• The motor is connected to the voltage source and is running at a constant speed. The back EMF has risen and the current has reduced from its starting value and is stable.
• If we now increase the voltage the current will increase. Remember that the current, \$ I = \frac {V_S - V_{bEMF}}{R} \$ where R is the motor resistance.
• The increased current will give increased torque which will accelerate the motor to its new steady speed.

But we know that speed is inversely proportional to armature current.

No. Speed is proportional to voltage. If you try to stall a motor running on constant voltage you will see the current rise. However, given a constant voltage the current will decrease as you approach the speed for that voltage. That may be the source of your confusion.

You could get a good feel for this if you have access to a lab power-supply with a voltage and current meter along with a suitable DC motor. Try running the motor in constant voltage mode an in constant current mode.

From the comments:

For a given load, an increase in voltage supply would increase the potential difference across the armature.

This is kind-of a redundant statement. For a permanent magnet motor the supply voltage is the armature voltage.

And this increase in armature p.d. generates a stronger magnetic field which interacts with the stator field to make the motor go faster.

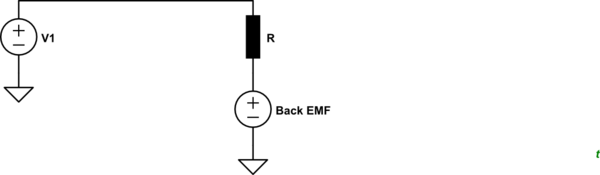
^{simulate this circuit – Schematic created using CircuitLab}

Try thinking of it like this:

• The motor acts as a generator. The faster it spins the more back-EMF it generates. Due to friction losses, etc. the back-EMF is less than the applied voltage.
• At startup a certain V1 is applied. The motor is stationary so the back-EMF is zero. A high current flows determined by V/R.
• The motor starts to spin up. The back-EMF rises. The current falls because R is no longer between V1 and 0 V but between V1 and V_bEMF. The faster the motor spins the more the back-EMF voltage rises and the current falls.
• The motor speed stabilises when the power in = the work it has to do.
• If we now increase V1 then more current will flow through R. This will provide more torque and accelerate the motor until a new stable operating point is found.