I don't understanding why we add these capacitors in the probe circuitry and make things more complicated.
They make things far less complicated. The low-pass filter that would otherwise occur is eliminated.
The point is to add enough impedance to a passive probe to minimize loading effect of the circuit being measured. A big enough resistor will do and the with just resistors, ...
No. Increasing the probe resistance decreases the high-pass cut-off as it will be proportional to \$ \frac 1 {RC} \$.
... voltage divider works the same for both AD and DC source regardless of frequencies.
No. You have omitted the effect of the scope's input capacitance.
If there are intrinsic reactances in the wiring, then can't we just add a big enough resistor in series with those to make any reactance negligible?
Increasing the resistance decreases the signal available to the 'scope to the point that you won't be able to get high enough resolution and ADC noise will become a problem.
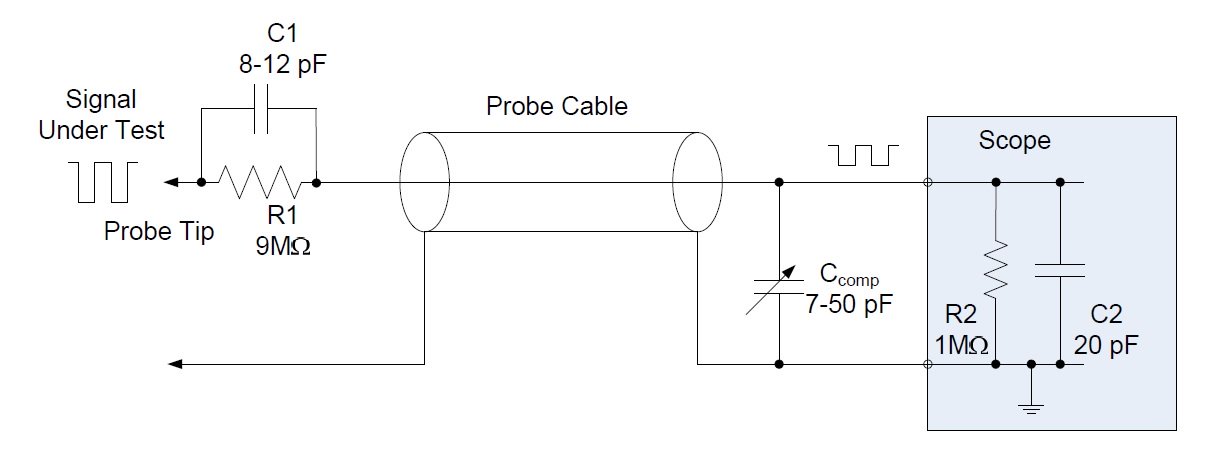
Figure 1. From Introduction to oscilloscope probes.
The trick is to use two potential dividers; one resistive and one capacitive. Since we've got a ratio of 9:1 with the resistive divider we need to do the same with the capacitive divider. Remember that the capacitors impedance is given by \$ Z_C = \frac 1 {2\pi fC} \$ so $$ \frac {R_1}{R_2} = \frac 9 1 = \frac {Z_C1}{Z_C2} = \frac {\frac 1 {2\pi fC_1}} {\frac 1 {2\pi fC_2}} = \frac {C_2}{C_1}$$$$ \frac {R_1}{R_2} = \frac 9 1 = \frac {Z_{C1}}{Z_{C2}} = \frac {\frac 1 {2\pi fC_1}} {\frac 1 {2\pi fC_2}} = \frac {C_2}{C_1}$$
From this we get \$ C_2 = 9C_1 \$. With the values shown in Figure 1 we can just about achieve this if C1 is 8 pF and CCOMP is wound up to the max to give us 72 pF total (although this model omits the cable capacitance so we have room to spare).