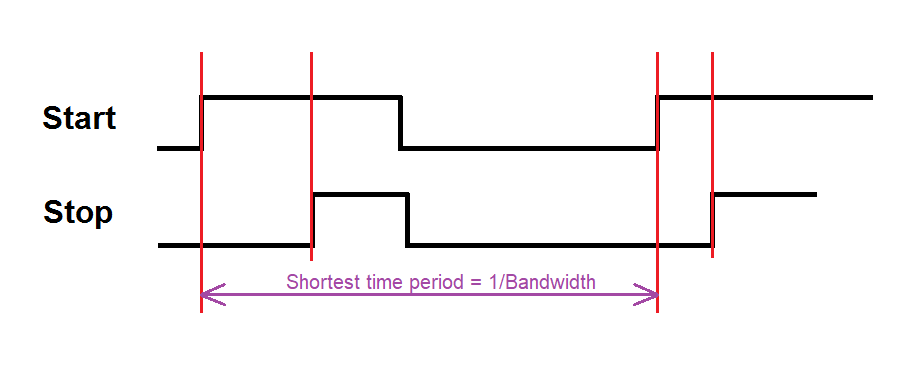
I see it like this: -
Or you could make an argument that the bandwidth is \$\dfrac{\text{shorted time period}}{2}\$\$\dfrac{\text{shortest time period}}{2}\$
This assumes that the longest time period is infinity. The longest time period defines the lower operating frequency of the bandwidth.
In other words, if you do not calculate the time difference for every occurance of a start/stop situation, the values will have the possibility of being aliased.
Hence it is the frequency of your stop (or start) signal that determines your bandwidth.