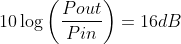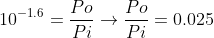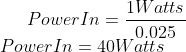Let´s supposeSuppose we have two arbitrary pointpoints A and B. Transmition trhoughtTransmission between these points are aboutis over optical-fiber. We don´t care the wavelenghtwavelength, distance and and and other stuffsstuff.
But now Let´sNow suppose we have total atenuationattenuation of 16 dB in average between these 2 points. According to general decibelsdecibel formula:
$$ 10\log_{10}\left(\frac{P_\text{out}}{P_\text{in}}\right) = 16\,\text{dB}$$
When we solve the formula and the relationship between Power Outpower out and Powerpower in :
$$10^{-1.6}=\frac{P_\text{out}}{P_\text{in}}=0.025$$
It means to the custumercustomer, just only arrives 0.025 of the power sent from dethe source (ISP, and so on) arrives. That means Ifif we want to the custumers arrivecustomers to receive 1 wattswatt, Wewe need to sent at least 40 watts:
$$P_\text{in} = \frac{1\,\text{W}}{0.025}=40\,\text{W}$$
My question is:
Does this really happen? Companies Do companies spend hundreshundreds of watts so that only a few watts reach customers because of attenuation? Does that make sense?