It may be worth slogging through the exact math to illustrate what's happening here. Assuming a source resistor \$R_S\$ and a load resistor \$R_L\$ the current through both resistors will simply be
$$I = V\frac{1}{R_S+R_L}$$
We can calculate the voltage over the load as a simple voltage divider $$V_L = V\frac{R_L}{R_S+R_L}$$
The power over the load becomes
$$P = I \cdot V_L= V^2\frac{R_L}{(R_S+R_L)^2}$$
If \$R_L\$ is very small the power becomes zero because the numerator becomes 0. If \$R_L\$ is very large the power also becomes zero since the \$R_L^2\$ term in the denominator dominates. In other words if \$R_L\$ is small the load voltage becomes zero and if \$R_L\$ is large, the load current becomes zero. In either case the product of the two becomes zero too.
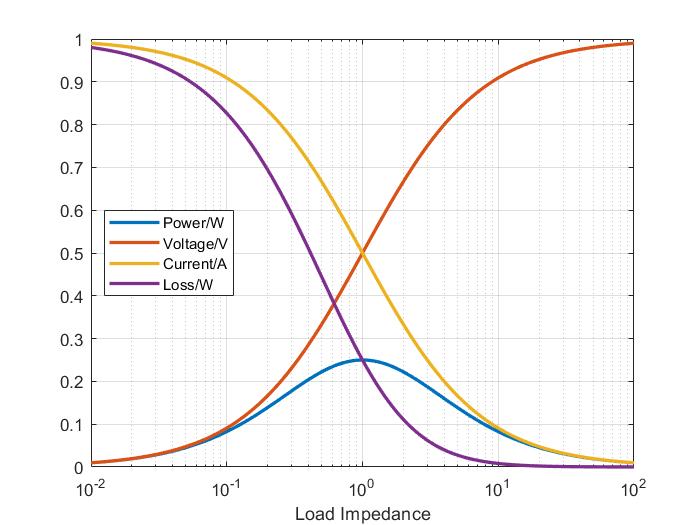
Let's take a look at a graph for a source of 1 Volt/1 Ohm:
The graph show power, voltage and current of the load as well as the "loss" which is the power consumed inside the source. Even though the source can deliver a maximum of 1 Watt, the max we can get into the load is 0.25 Watts. At this point the power in the source and the load is the same, so the efficiency is 50%.
To maximize the power, we need maximized this function, i.e. solve for
$$\frac{\partial P}{\partial R_L} = 0$$
Unfortunately that's bit of a slog. Using the quotient rule, we get
$$0 = \frac{\partial P}{\partial R_L} = V^2\frac{(R_S+R_L)^2-2R_L(R_S+R_L)}{(R_S+R_L)^4} $$
We can dump the constant multipliers and get:
$$0 = (R_S+R_L)^2-2R_L(R_S+R_L) = R_S^2+2R_SR_L+R_L^2-2R_LR_S-2R_L^2 = R_S^2-R_L^2$$
Technically this has two solutions
$$R_L = \pm R_S$$
but if only allow positive resistances, the only solution is
$$R_L = R_S$$
If we would allow for complex impedances, we would get \$Z_L = Z_S^*\$, i.e. load and source impedance have the same magnitude but opposite phases. We leave this derivation for another day :-)