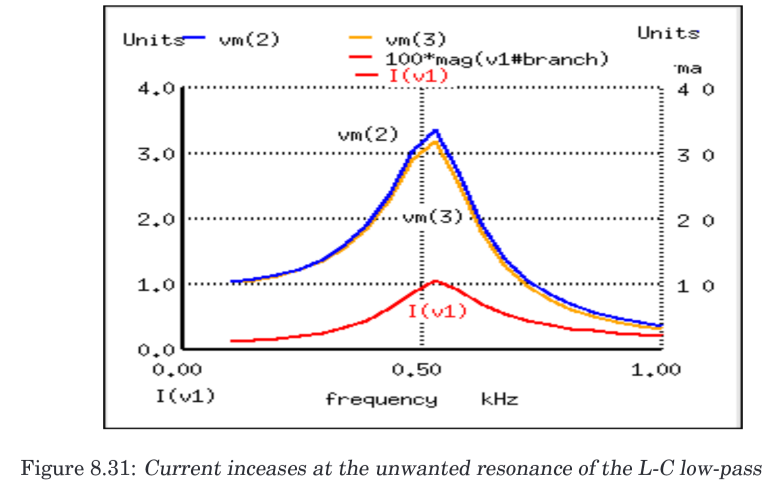
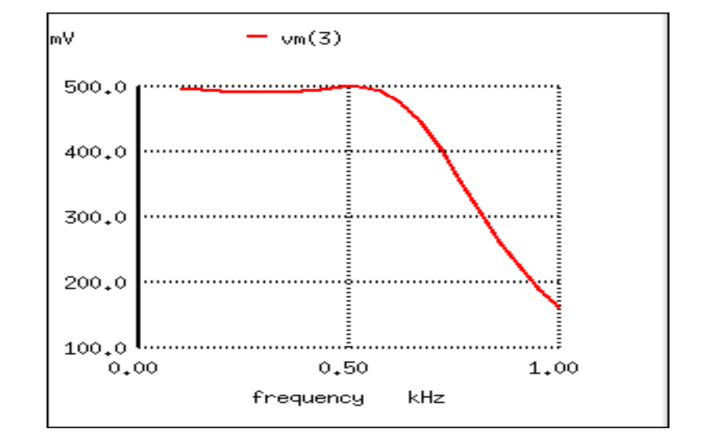
The author of the textbook containing this circuit states that the filter input and output impedance is given by:
Z = (L/C)^1/2
He then shows that by setting the source and load impedance to this value (316 ohms), we can "flatten" the frequency response of this filter.
But thisThis leaves me with three questions:
- Why does impedance matching flatten the response?
- Where does this input / output impedance equation come from?
- Why doesn't the equation account for frequency, since the reactance of capacitors and inductors varies with frequency?
Thanks for your time.
Also, before it is pointed out, I know this is the second post about a really simple circuit. But, theseThese three questions are new and I have not been able to answer them on my own, despite a lot of time invested.