This is basic feedback stuff.
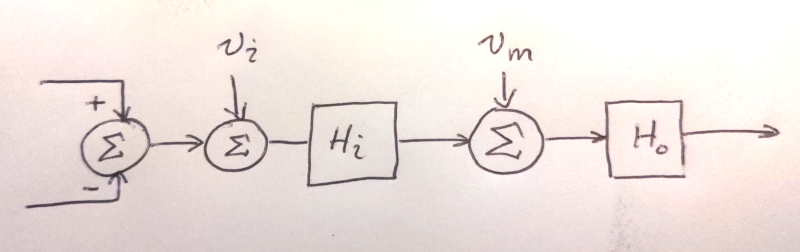
The diagram shows a model of an op-amp that shows just the parts of the problem that concern us here. \$v_i\$ is the "usual" first-stage input offset that you're thinking about. \$H_i\$ is the first-stage gain (your "intrinsic gain"), \$v_m\$ is the offset voltage of the intermediate stage, and \$H_o\$ is the gain of the rest of the op-amp.
For the moment, consider \$v_i\$ to be zero. Then, for the output to be zero, \$v_m\$ must be overcome. For that to happen, the input differential voltage must be equal to $$\frac{v_m}{H_i} \tag 1$$.
If by "intrinsic gain" you mean the transistor's intrinsic gain, \$g_m r_o\$, then your \$H_i\$ will, at DC, roughly equal \$H_i \simeq g_m \frac{r_o}{2}\$*, or half of the \$g_m r_o\$ product.
That is why the intrinsic gain matters. Look at (1) and ask what happens as \$H_i \to \infty\$. Now look at (1) and ask what happens as \$H_i \to 1\$. Your question should be answered.
* Analyze the circuit behavior around M1-M4. It should be clear. Note that I may be off by a factor of 2 or 4, but the gain of that stage is -- to a first order -- going to be proportional to \$g_m r_o\$.