Simple: the magnetizing inductance doesn't play much role in the LL-Cs resonance. Core loss is effectively in parallel equivalent with the magnetizing current (primary, i.e. the primary or secondary inductance). If the magnetizing current is small enough -- you really don't care what phase it has!
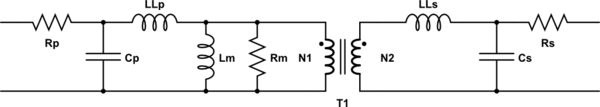
simulate this circuit – Schematic created using CircuitLab
This is a typical 2nd order nonideal transformer model. Rp/Rs model DC resistances, Cp/Cs the total winding self-capacitances (there will also be some across the isolation barrier, between the respective ends of the windings), LLp/LLs the leakage inductance (for \$k \rightarrow 1\$, we can approximate it by lumping it all to one side; at lower \$k\$, we might want to use both), Lm the magnetizing inductance, and Rm its loss. T1 is an ideal transformer with ratio N2/N1.
As a first order model, core loss can be represented simply as a resistance in parallel with the magnetizing inductance.
This approach is also the trick with RF transformers: it's hard to find any material that's meaningfully inductive (not loss dominant) at 100s of MHz, but simply using enough turns that the magnetizing impedance is several times the system impedance (i.e., say 200 ohms compared to the 50 ohm transmission line it connects to) gets you there with <1dB insertion loss, and if that's good enough, who cares, eh?
It may be worth noting that fringing fields and leakage flux (the flux literally leaking out of the core, into space -- not necessarily just that measured between windings) flow thru-plane in these materials (high-mu amorphous/nano. materials are made in strip form only), so will encounter high losses. This is really only a problem with uneven windings, or around air gaps.
Uneven windings includes the sectoral wind on a toroid, such as commonly used for common mode chokes. In that case, the differential mode will have higher losses. (But, that's not at all a problem in that application; it might be valuable if anything.)
These materials are also available in cut C cores, which can be air-gapped for inductor use (energy storage). This isn't useful at high frequencies for this reason; but the low losses at mains frequency may still make them useful (if a rather expensive choice).
