The answer lies in Kirchhoff's Current Law (KCL), from which we can state that within a closed circuit, the current is equal at each point in the circuit. The current that flows in is equal to the current that flows out.
Yes, kind of like water into and out of a garden hose. Push current in, the same current pushes out. What will differ is the pressure at each end. Back in the electrical world, voltage is pressure, while current is flow.
Consider an LED in series with resistor, connected to a battery. That's a closed circuit. KCL tells us that LED and resistor will see the same current, regardless of connection order.
Now, each element has its own voltage drop.
- LED voltage drop is its forward threshold voltage (Vf)
- Resistor voltage drop is proportional to current (E = I*R).
The total voltage drop for the two will be Vf(LED) + I*R.
Notice that '+' sign. You may recall that addition is commutative. It doesn't matter what order you do the addition. You get the same total voltage drop.
Further, regardless of connection order, because we know based on KCL that the currents will be the same, so will the terminal-to-terminal voltage drop of each. Vf will not change, nor will E = I*R. The only difference will be the voltage seen between the resistor and LED relative to the power supply.
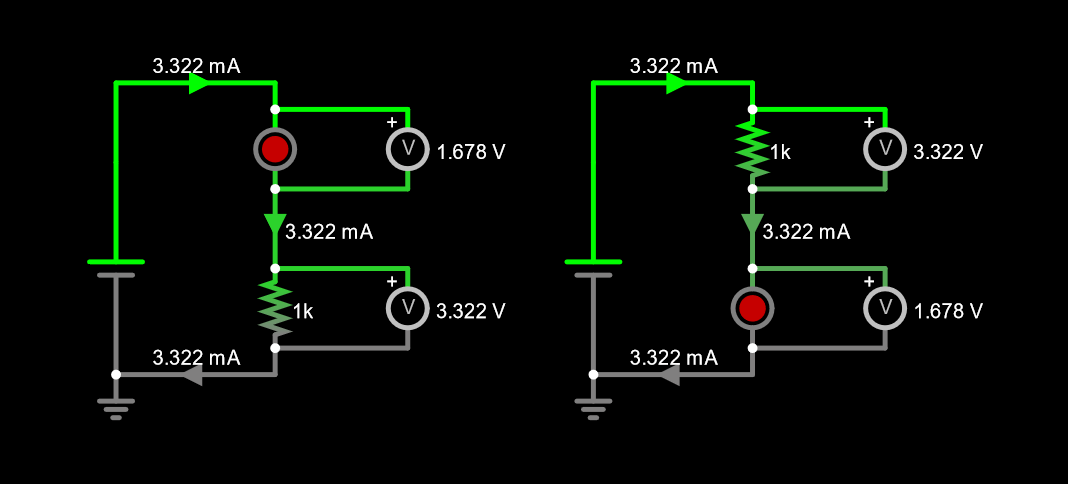
Here's a quick sim to show what's up (simulate it here):
You'll notice in each case that:
- LED forward drop is the same
- Resistor IR drop is the same
- The currents are equal everywhere
The only difference we see is the voltage between the LED and resistor, since that shifts with the component order.