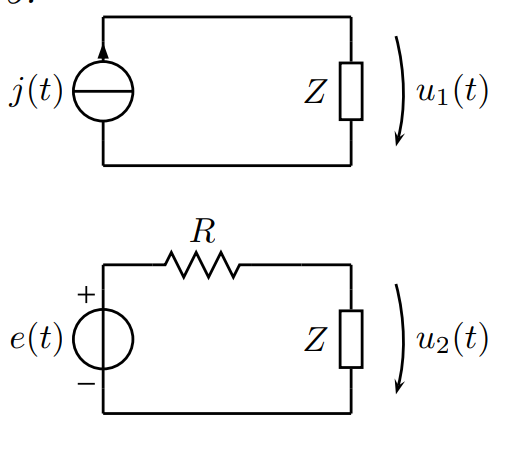
Goal is to solve for the voltage u2u2(t)
Okay so I'm some whatsomewhat confused onby this. I solved the transfer function \$Z(s)\$Z(s) earlier in the top circuit, which is simply just Z. The voltage across Z in the top circuit is $$u_1(t)=5e^{(-100*t)}u(t)$$$$u_1(t)=5e^{(-100t)}u(t)$$ and the current source is j(t)=u = u(t), where u(t) is the step function. Z is a circuit system itself with no independent sources and all initial values are zero.
Using basic principles for u2u2(t) you obtain e(t)*Z·Z/(R+Z), where again e(t)=u = u(t), so it's u(t)*Z·Z/(R+Z).
Obviously, just from that equation you cannotcan't solve thefor voltage. I'm thinking this has something to do with the frequency response, but I don't know how I'm supposed to use it for this problem.
ZsZ(s) is the same in both circuits are the same.