I'm only going to answer one of your questions. It would be better if you asked your questions separately.
My last is, how does one know what the maximum frequency that one can put into an op-amp is?
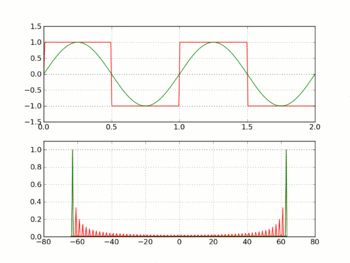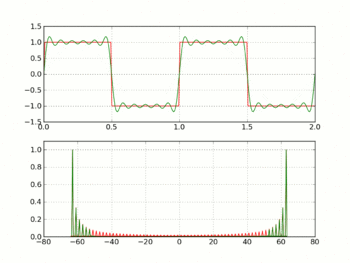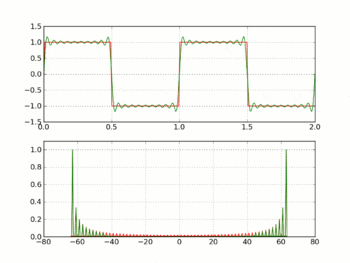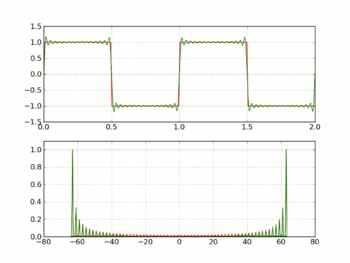
This is specified in the datasheet. Applying a frequency beyond the op-amp's specifications won't harm it. The limitation is simply that as frequency increases, the op-amp's gain decreases. Essentially, it is a low-pass filter. The image from your question illustrates this:

If your input square wave is very much below the op-amp's cutoff frequency, then it will pass through unchanged. As the cutoff frequency gets closer to the square wave frequency, it begins attenuating the higher harmonics, which has the effect of increasing the rise and fall times. Eventually, all the harmonics will be attenuated, and the square wave will start looking more like a sine wave. As the square wave frequency increases beyond the op-amp's cutoff frequency, the entire signal is attenuated, until you are left with nothing at all.
Here's an animation from Wikipedia showing the shape of the square wave as higher harmonics are added. The process I described is the same thing, but in reverse: the higher harmonics are attenuated.