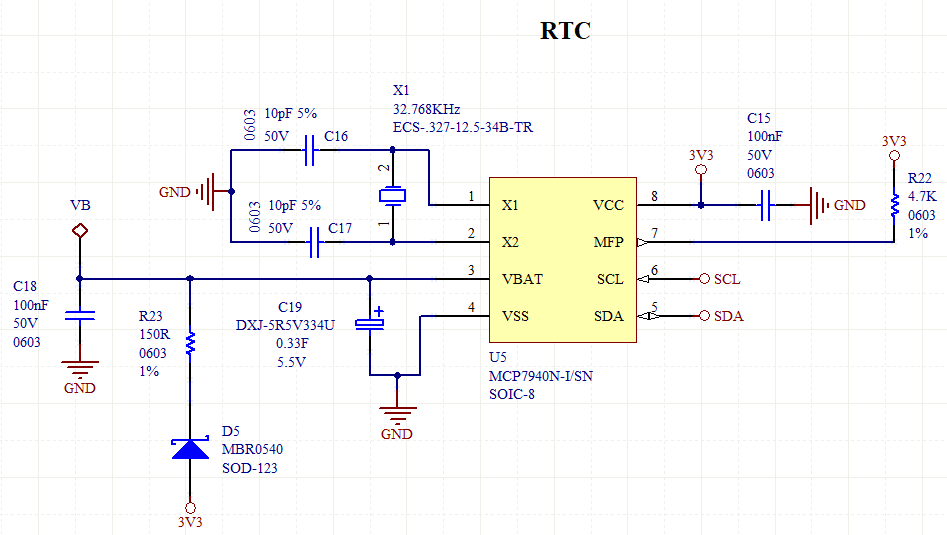
This is my current RTC circuit...
Supercapacitor = DXJ-5R5V334U (ELNA)... DXJ-5R5V334U = 0.33F, 5.5V, ESR = 150 Ohms Resistor = 150 ohms 0603 100mW 1%
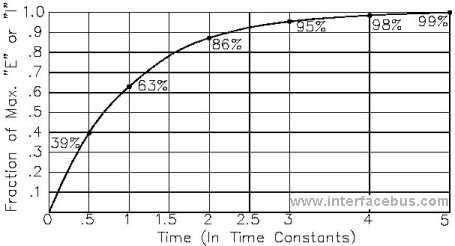
If I consider only the resistor, I have a RC constant of 0.33 * 150 = 49.5 (~50) seconds... 50 seconds x 3 = 150 seconds (3x RC = capacitor very well charged, 95%...). That is 150 seconds... divided by 60 = 2,5 minutes...
But if I consider also the ESR of the supercapacitor, I will have 300 Ohms in total, so that the charging time would be 5 minutes, double the time...
What value of resistance should I consider in practice? 150 ohms (2.5 minutes)? Or 300 ohms (5 minutes)?
Another question related to that, is: the VBAT pin consumption is 1.2uA (@ 3.3V)... If the supercapacitor is fully charged (like 95%) and the circuit loss the power supply of 3.3V (shutdown/off), the RTC will continue to operate from the VBAT pin (supercapacitor), so how much time the RTC would stay working from VBAT? (1 month? more?). As I said, consumption is 1.2uA (@ 3.3V) and the capacitor is 0.33F charged with something like 3 to 3.1V, how much time the RTC IC would stay working if the 3.3V rail keeps off "foreve"r? According the the datasheet of RTC chip, the "Backup supply voltage" minimum voltage is 1.3V. Do you know some online calculator where I could estimate that time of operating from VBAT pin after power-off of the board and capacitor being 95% charged? Could you calculate these things to me please? Or maybe an online calculator would be great also...
Thanks and Best Regards...