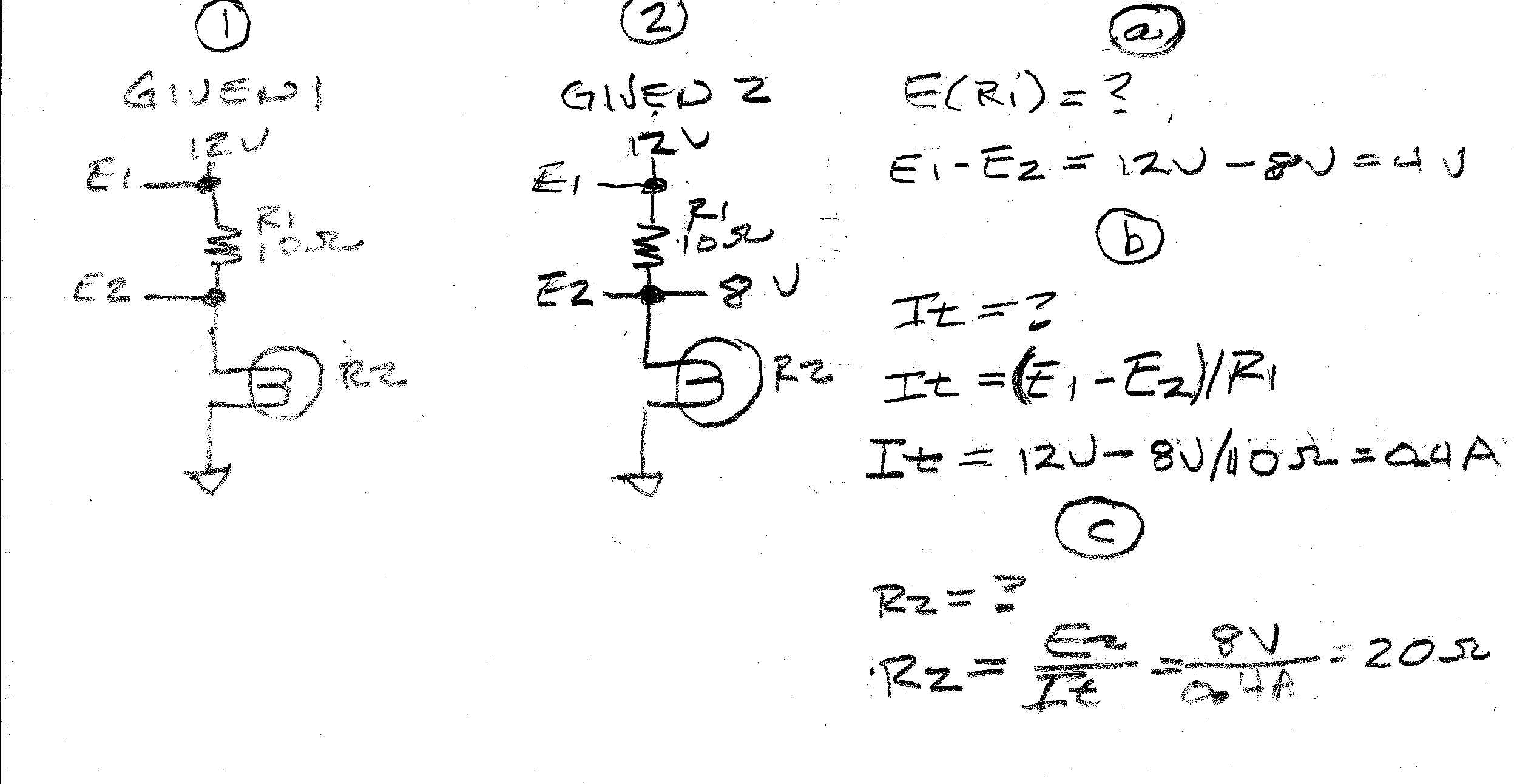Ohm's Law and in-series components

Question

I am doing some practice problems based on what I learned today in lecture. However, I am stuck on this problem:

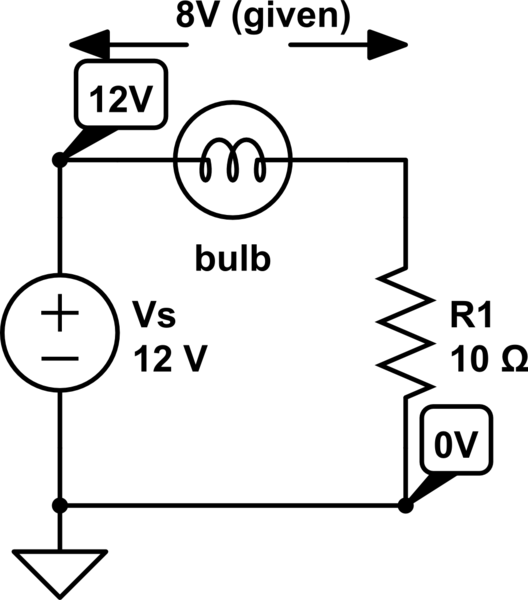
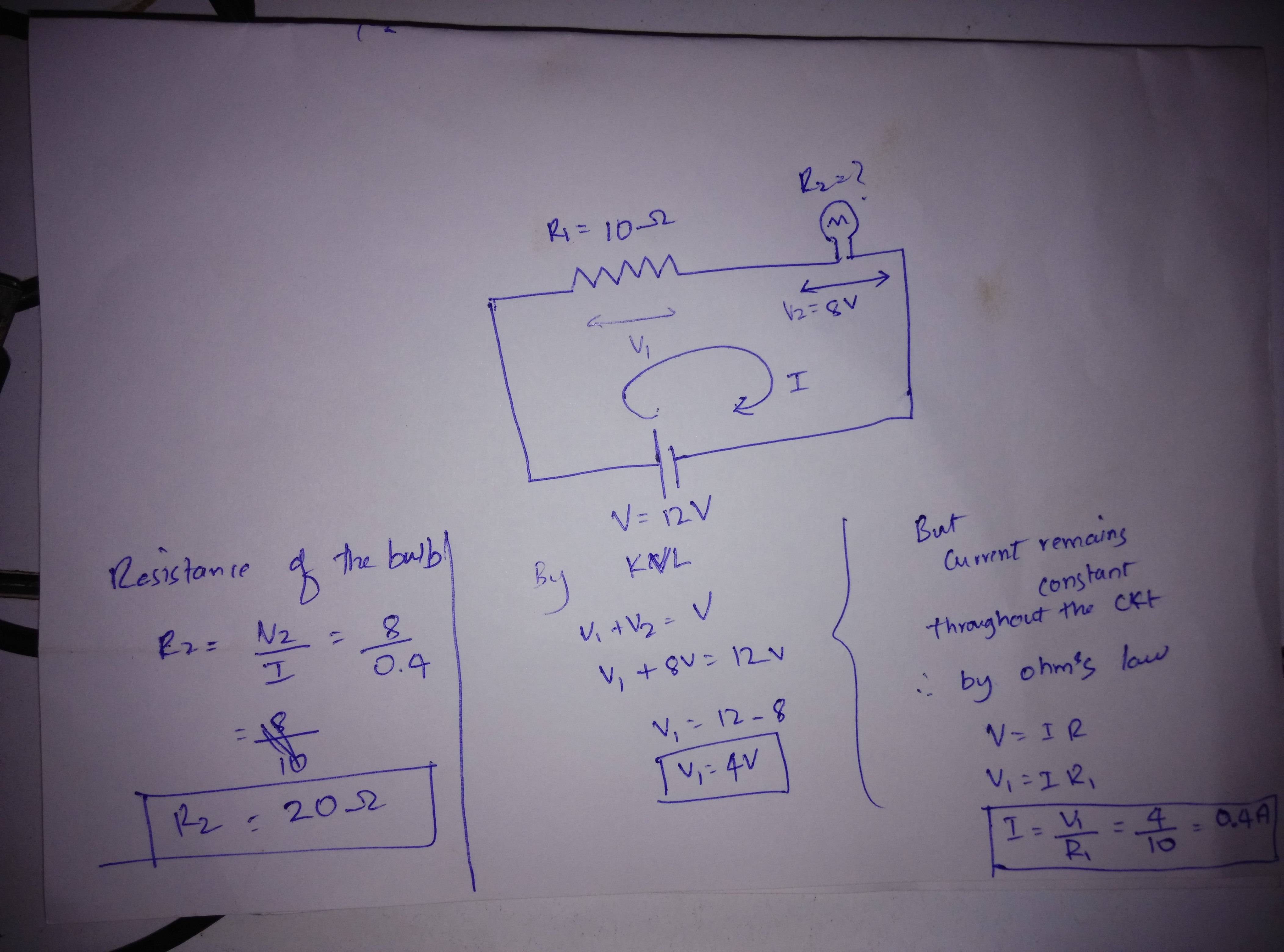
A 10Ω resistor is in series with a bulb and a 12V source.

a. If 8V is across the bulb, what is across the resistor? b. What is the current in the circuit? c. What is the resistance of the bulb?

Where would I begin solving what voltage is across the resistor? I do not the current, nor do I have the means to find it (that I can see), thus I can not find what voltage is dropped. I am not sure if I can assume the bulb has no resistance, in which case I can solve the problem easily.

Answer 1

You need to use KVL around the loop:

^{simulate this circuit – Schematic created using CircuitLab}

KVL says that the sum of the voltages around the loop equals 0. That means that if there is a 12V increase traveling from the negative to the positive terminal of the voltage source, then the voltage must fall by 12V traveling through the bulb and resistor.

The current in the loop can be found using Ohm's Law and from the voltage \$V\$ across the \$10\Omega\$ resistor you found in part (a).

The resistance of the bulb can then be found using Ohm's Law again: you are given the fact that 8V is across the bulb and from part (b) you know the current through it. Solve for the resistance \$R\$.

Answer 2

Just remember that Voltage will divide/split across the resistors when they are connected in series(Note: Sum of voltage across all the resistors are equal to applied voltage(by KVL)) but the current remains constant in all the resistors. Similarly current divides across the resistors when they are connected in parallel(Algebric sum of current entering and leaving the node is equal to 0(by KCL)) but voltage remains constant across the resistors..

Solution for your problem,

Answer 3

Considering the bulb as having reached equilibrium, this should do it:

Answer 4

The voltage total has to equal 12 volts unles they are lying about it then you take the voltage youself butthis is theoretical so it is 12 volts. Subtract the voltage drop you know and it gives the voltage you dont know. My math is not the greatest but a little kid could give you this counting on fingers 4 volts then your the college person that knows how to appie ohms law. So you have resistance and voltage you find current you can get wattage. If the lightbulb is unenergized it has a differeent resistance than in an energized state. That has nothing to do with the anwer just screwing with you. If the instructor gives you a circuit with a whole page of this with 21 elements its the same type of problem dont get nervous on the test just plug thru it finding each thing you can but dont make math mistake like 2 and 2 is five.