Image of surface, shown in blue, through which magnetic flux passes, indicating the boundary in red, in motion at velocity v:
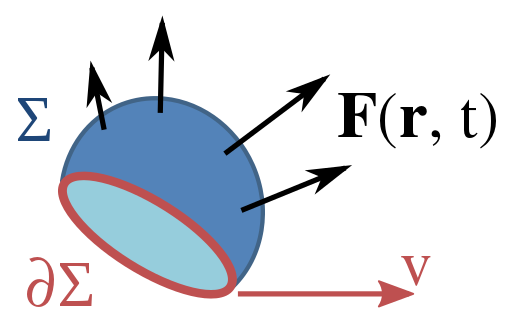
In the Wikipedia article on Magnetic Flux, it is said that an EMF is induced around the boundary of an open surface transiting a magnetic field such that the magnetic flux is normal to that surface. An accompanying diagram shown here explains the various relations, indicating the surface boundary in red and showing that such flux is generally aligned with the B-field in this case.
By Faraday's Law, the effect in which EMF arises is described as the work required to move a charge along that boundary, in combination with the motion of the boundary (including its changes in orientation).
So, in a simple 2-pole scenario of EM induction in which a copper loop is rotated through respective B-fields of the North and South poles, what is the disposition or orientation of such a surface and its boundary with respect to the cylindrical geometry of the copper conductor? This is the main question being asked here. In other words, is the direction of motion of the boundary at velocity v generally that of the rotating loop?
Secondarily, if for instance the surface of that geometrically cylindrical conductor exposed to the 'magnetic flux' arising from one pole is akin to that shown in the diagram so that the boundary is an ovoid (with its long axis in the longitudinal direction of the conductor) around which an electron is moved by the magnetic force implicit in that field, then is that evidently oscillatory motion of the charge/electron around that boundary the basis of AC?
What then is its physical relation to a corresponding surface and boundary simultaneously transiting the other pole? And would it then be generally correct to describe that relation between remote effects in the conductor at the two poles in terms of a phase relation between respective 'electron wave functions', for example by the inference of a phase factor in the exponent of that function?

what is the disposition of such a surface and its boundary with respect to the cylindrical geometry of the conduit?What are you asking here, by "disposition" do you mean what outlines the shape? Or where the shape is, how it's shaped in general? (As it happens, the field equations don't care where the surface is, given certain continuity etc. conditions; its perimeter is sufficient information.) What do you mean by "cylindrical geometry"? It's a loop of wire, not a cylinder. Do you mean the (generally cylindrical, albeit bent into a loop) shape of the wire itself? \$\endgroup\$