DCM vs CCM Noise Spectrum

Question

Will the noise spectrum (at the LISN) due to switching of a Synchronous Buck DC-DC converter be different (peaks) if the converter is operating in DCM (Inductor current) compared to CCM keeping the converter same. CCM to DCM I did by increasing the load resistance.

Answer 1

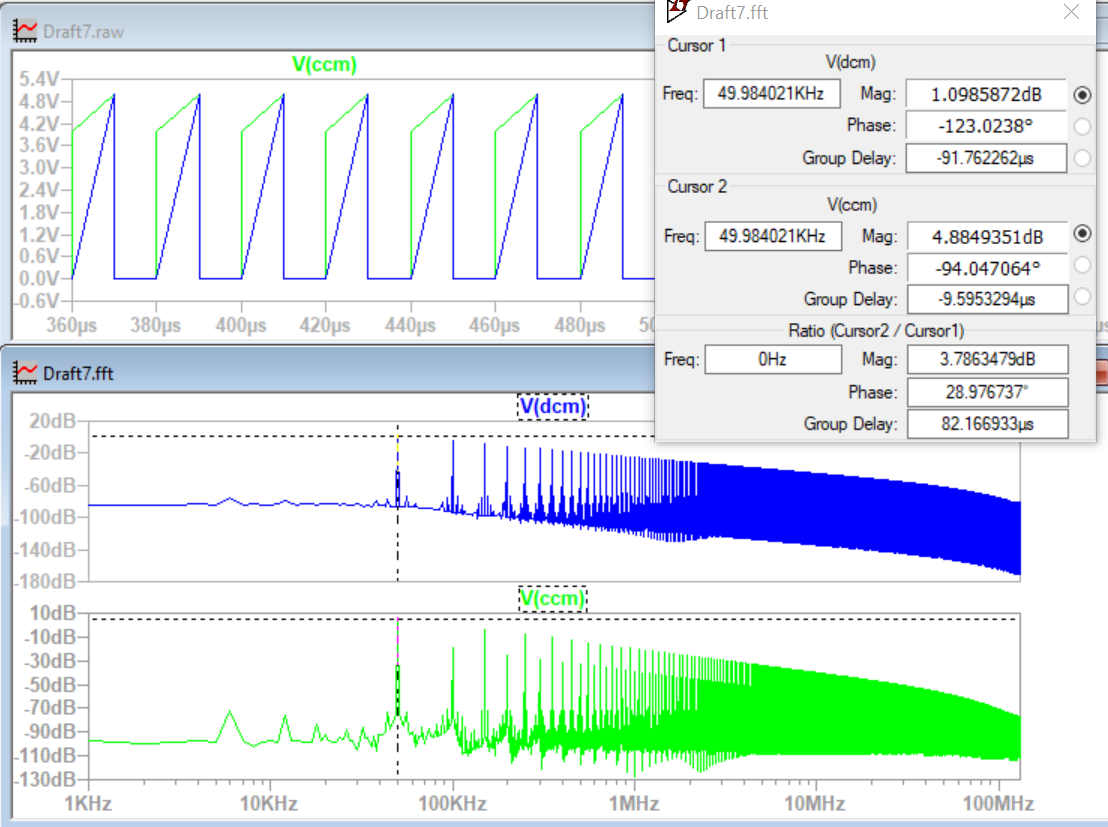
Here is a really quick and dirty simulation for you. Several downsides: no filters what so ever and would not transfer the same amount of power. Just the top switch waveform difference.

Answer 2

In a fixed frequency DC-DC converter, the components of the spectrum will always be rational multiples of the switching frequency. However, the amplitudes at each rational multiple of the switching frequency will depend upon the operating conditions of the converter. Changing between DCM and CCM will change the relative amplitudes of the components. However, even changing the duty ratio of the switch will change the amplitudes as well.

To a first approximation, the voltages and currents in a DC-DC converter are periodic with the switching frequency. Each periodic function has a Fourier series which (with a slight lie) is equal to that periodic function. All of the components of that Fourier series are multiples of the fundamental frequency. To a first approximation that fundamental frequency is the switching frequency.

To make matters slightly more refined, DC-DC converters may exhibit sub-multiple oscillations. For example, switching cycles may alternate between two different pulse widths. Or every third pulse may be skipped, etc. So, the fundamental frequency for a Fourier series may not be the switching frequency, but some submultiple of that frequency. (I suppose it is also possible that it be an irrational multiple of that frequency, but we usually don't extend our analysis that far).

In any event, changing the current or voltage waveform, doesn't change which frequencies are rational multiples of the switching frequency, but it will change the amplitudes (and phases!) of the various Fourier components.

[The little lie about Fourier series, is that the limit of the series does not always match the original waveform if the original waveform has jump discontinuities -- the reconstituted waveform may have "spikes". For example, the limit of the Fourier series for an exact square wave is not exactly a square wave, but a square wave with spikes at each transition. However, with a sufficient number of terms, the spikes become arbitrarily "narrow", but not arbitrarily small in amplitude". This effect is known as the Gibbs Phenomenon.]