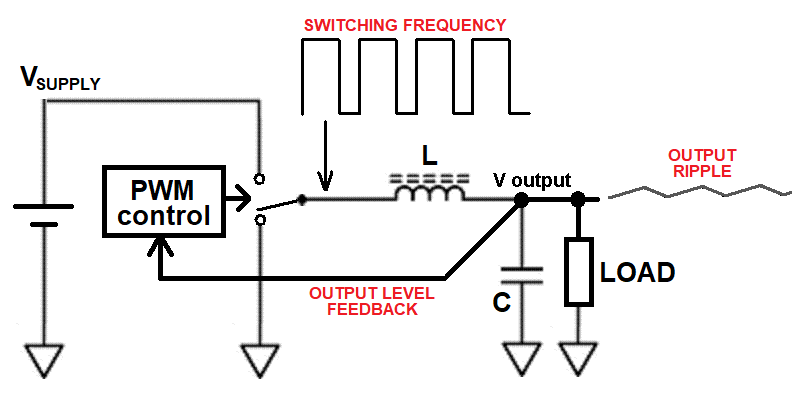
Which cross-over frequency is this referring to with respect to the DC-DC Converter Switching frequency
It's more than likely referring to the the LC resonant frequency (\$f_c\$) of the energy storage components within the DC-to-DC converter. See L and C below: -
Cross over frequency should not be higher than 1/8th of switching frequency
The L and C form a low pass filter that below resonance, barely alter the phase angle between input (the switching waveform) and output (the smoothed DC voltage). However, as resonance (\$f_c\$) approaches, the phase changes dramatically from near 0° to 180°. That change of phase is unavoidable and can turn a stable circuit into an unstable oscillator. \$f_c\$ has to be some way below the switching frequency for it to be effective.
Simulation
Using an on-line simulator, consider L = 10 uH and C = 10 uF for the energy transfer components and, look at the green trace (phase response) below: -
Link to Interactive calculator
Slightly below \$f_c\$ =15.9 kHz (referred to as \$f_n\$ in the picture above), the phase shift is quite close to 0° and this poses no threat of introducing loop instability. However, slightly above 15.9 kHz, the phase has shifted nearly 180° and this can really "shake the ground" when it comes to stability. This is why compensation circuits are added within the PWM control block (see top picture) to retard the 180° phase shift and prevent this oscillatory condition arising. The compensation is a counter-measure to unwanted oscillation.
To achieve adequate filtering of switching voltages on the output, you need to keep the resonant frequency (\$f_c\$) of the energy transfer components (L and C) significantly below the switching frequency. The further you go below the switching frequency, the better the result i.e. lower output ripple voltage. The LC is a great low pass filter for this and, in the above picture, you can probably see that if the switching frequency were at 159 kHz (\$10\times f_c\$), the attenuation of the switching voltage will be 40 dB compared to DC. That's a 100:1 reduction
Example: if the switching is 10 volts p-p, the resulting 1st harmonic on the output waveform will be 100 times lower at 100 mV p-p. However, you also want to keep the resonant frequency high so that your closed-loop control system can react quickly to load and supply changes.
These two requirements are in opposition so a compromise is necessary.
Why 8:1? Why not 10:1? It's a rule of thumb and like most rules of thumb, you can choose to push the rule this way or that way depending on your most dominant needs.
Hopefully, the information above will allow you to see that the choice of LC cross-over frequency is a compromise based on juggling these somewhat opposing constraints: -
- Good loop response to load and supply voltage changes (\$f_c\$ needs to be high)
- Ensuring the compensation circuit is effective at the resonance (\$f_c\$ "right")
- Minimizing output ripple voltage (\$f_c\$ needs to be low)