I have found that a commonly mentioned definition for the resonance frequency in an RLC circuit is defined as the condition where the capacitive and inductive reactances cancel each other, resulting in a purely resistive impedance.
However, though the simplest RLC circuits (e.g RLC all in series or parallel) show maximum/minimum current at the resonance frequency defined above, this is not generally the case. For example, the RL-C series-parallel circuit
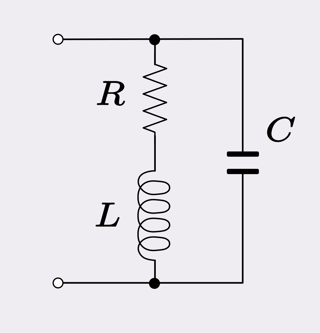
has a resonance frequency at $$\omega_0 = \frac{1}{\sqrt{LC}}\tag{1}$$ by the above definition, but shows a minimum current at $$\omega_m = \sqrt{\sqrt{\frac{1}{L^2C^2}+\frac{2R^2}{L^3C}}-\frac{R^2}{L^2}}\tag{2}$$ My question is why is it defined this way? Would it not be more practical to define it as being at the maximum/minimum magnitude of impedance so that the observables (current and voltage) are also at extrema?
